2.2.6 Calculating U-values of multiple layers of materials
Any thorough analysis of the thickness of insulation required to meet a specified U-value will require some detailed calculations. The earlier discussion of the basics of U-values only considered the thermal resistance of a single slab of a building material.
In any practical building element there will be extra thermal resistances, particularly those of the thin layers of air adhering to the outermost and innermost layers of the material, and the air in any substantial gap between the layers. Table 5 gives standard thermal values used for these. Note that the outside surface resistance is much lower than the value used for the inside surface. This is because the air is less likely to be still on the outside and will thus provide a relatively poorer insulation performance.
| Layer | Resistance / m2 K W–1 |
|---|---|
| Inside surface (Rsi) | 0.13 |
| Air gap | 0.18 |
| Outside surface (Rso) | 0.04 |
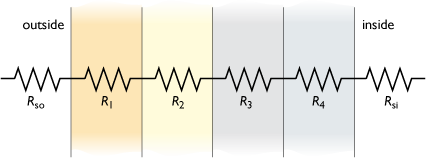
The thermal resistances of the components of a building element can be added in series as in Figure 16, to give a total thermal resistance (rather like adding electrical resistances in series). The total thermal resistance of a practical building element will thus consist of the sum of those of all its layers plus the inside and outside surface resistances.
Taking, for example, a wall construction with four layers, the total thermal resistance, RT , will be:
- RT = Rso + R1 + R2 + R3 + R4 + Rsi m2 K W–1
The U-value of this wall is its inverse = 1/RT W m–2 K–1
For example the wall shown in Figure 15 consists of the following layers: 115 mm common brick, a 115 mm cavity filled with mineral wool (conductivity 0.035 W m–1 K–1), 115 mm of aerated concrete blockwork (density 460 kg m–3) and a 13 mm layer of plaster on the inside. Using the conductivity values in Table 4 we can calculate its U-value by summing the various thermal resistances as shown in Table 6.
| Layer | Thickness /m |
Conductivity/ W m–1 K–1 |
Resistance/ m2 K W–1 |
|---|---|---|---|
| Outside thermal resistance | 0.04 | ||
| Brick | 115 mm | 0.77 | 0.115/0.77 = 0.15 |
| Mineral wool | 115 mm | 0.035 | 0.115/0.035 = 3.29 |
| Aerated concrete block | 115 mm | 0.11 | 0.115/0.11 = 1.05 |
| Dense plaster | 13 mm | 0.57 | 0.013/0.57 = 0.02 |
| Inside thermal resistance | 0.13 | ||
| Total thermal resistance | 4.67 |
The overall U-value is then:
- U = 1 / R = 1 / 4.67 = 0.21 W m–2 K–1
In practice, building elements do not simply consist of flat layers. The wall construction above is likely to use thin metal wall ties securing the outer brickwork to the inner leaf of blockwork. This will create a ‘thermal bridge’ bypassing the insulation and reducing its performance. Depending on the details a more realistic U-value for this sort of construction might be about 0.25 W m–2 K–1.
Similarly, in Figure 10, the base layer of loft insulation only blocks the flow of heat over a certain area. There is a parallel heat-flow path through the wood of the joists supporting the ceiling. This flow is blocked by the top insulation layer. A certain allowance always has to be made for thermal bridges, but the mathematics is not simple.
Activity 5
Ignoring the thermal resistance of the panes of glass, use the data in Table 5 to estimate the U-value of a double-glazed window.
Answer
The total thermal resistance of the window is the sum of the resistances of the inside layer, the air gap between the panes and the outside surface layer.
- Total resistance = 0.13 + 0.18 + 0.04 = 0.35 m2 K W–1
- U = 1/R = 1/0.35 = 2.86 W m–2 K–1
This answer is very close to the value of 2.7 W m–2 K–1 given in Table 2 for air-filled double-glazing, though this also takes the heat loss through the window frame into account.
Activity 6
What is the thermal resistance of a 4 mm thick sheet of window glass? (You will need to look back to Table 3 in Section 2.2.3.) Is doubling the thickness of the glass likely to improve significantly the overall U-value of a double-glazed window?
Answer
Table 3 gives the conductivity of glass as 1.05 W m−1 K−1. The thermal resistance of a 4 mm thickness will thus be only 0.004/1.05 = 0.0038 m2 K W−1. This is only about 1% of the calculated total thermal resistance of the window in Activity 5. Doubling the thickness of the glass will double its thermal resistance but won’t make much difference to the overall window U-value.
Activity 7
(a) Exploring the improvement in U-value resulting from the introduction of cavity wall construction
Table 6 above shows a calculation of the U-value of a modern multi-layer wall. A normal pre-1919 UK house is likely to have solid walls two bricks thick, with each brick being 115 mm thick (see Figure 12(a)). Later construction used cavity walls with an air gap between the two skins of brick as illustrated in Figure 12(b).
Table 7 is interactive and allows you to change the wall construction in the third layer giving three options:
- a solid brick wall two bricks thick
- a cavity wall
- a solid brick wall three bricks thick.
The overall calculated U-value appears at the bottom.
Which of the following gives the lower U-value?
- i.adding a cavity to a two-brick solid wall
or
- ii.increasing the thickness of the solid wall to three bricks thickness?
Table 7
(b) Exploring the benefits of cavity wall insulation and the thickness of insulation needed to meet future UK U-value standards
The interactive Table 8 allows you to calculate the U-value of a cavity wall filled with insulation (as shown in Figure 15). It also allows you to change the inner leaf between brick and aerated concrete. (Note that you will need to click on the ‘calculate’ button to produce the answer at the bottom.)
Table 8
- i.Start by calculating the U-value of a cavity wall with a brick outer skin in layer 2, a brick inner skin in layer 4 and insulation in a 50 mm cavity. A typical conductivity value to use for blown mineral wool cavity insulation might be 0.035 W m−1 K−1. The properties of other types of insulation have been given in Table 4. This should give a U-value of 0.52 W m-2 K−1. How does this compare to the U-value of the uninsulated cavity wall in part (a) of this activity?
- ii.Next explore the improvement in U-value by changing the inner leaf of the wall to insulating aerated concrete in layer 4. Remember to click on ‘calculate’ to produce the final U-value.
- iii.Increase the thickness of the insulated cavity to 100 mm or 150 mm. What is the U-value now?
- iv.Future UK houses may need walls with a U-value of 0.15 W m-2 K−1 or better. What is the minimum insulation thickness they will need using mineral wool? What is the answer if they used polyisocyanurate foam with a conductivity of 0.023 W m−1 K−1?
Answer
(a)
- i.Adding an air gap to produce a cavity wall decreases the U-value from 2.03 W m-2 K−1 to 1.49 W m-2 K−1.
- ii.Increasing the thickness of the solid wall to three bricks thickness reduces the U-value to 1.56 W m-2 K−1.
The cavity wall gives the greater decrease in U-value.
(b)
- i.Filling the cavity with mineral wool insulation reduces the U-value from 1.49 to 0.52 W m-2 K−1, almost a three-fold improvement.
- ii.Changing the inner leaf from brick to aerated concrete improves it further to 0.36 W m-2 K−1.
- iii.Increasing the insulation thickness to 100 mm improves the U-value to 0.24 W m-2 K−1 and 150 mm gives 0.18 W m-2 K−1.
- iv.The minimum cavity thickness to achieve a U-value of 0.15 W m-2 K−1 with mineral wool is 180 mm. This figure comes down to only 120 mm if polyisocyanurate foam is used.