1.7 Scientific notation
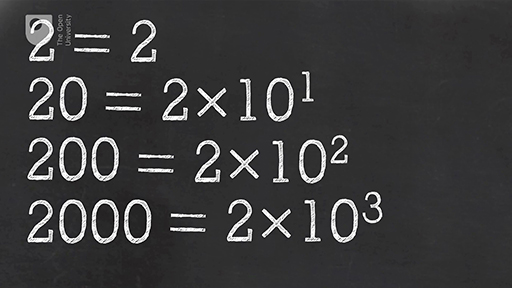
Transcript
Activity _unit1.7.1 Activity 1.3 Trying out scientific notation
This video introduces scientific notation – the way scientists write very big and very small numbers. Try to think of large or small science-related things and practise writing them in scientific notation by posting them in the forum thread for this activity [Tip: hold Ctrl and click a link to open it in a new tab. (Hide tip)] .
There’s an additional challenge in this activity … how do you write the superscripts? When you compose your post, you should see a button which looks like this: X2. To make a number superscript, highlight it using your mouse and then press this button.
Try it for yourself by posting a large number using scientific notation.
