3.2 Always, sometimes, never true?
As previously discussed, algebraic expressions are like mathematical phrases or sentences. Equations are like mathematical statements. A mathematician will always ask whether or not an equation is true.
When equations contain no unknown quantities, use calculation to check if they are true:
is an equation but it is not true.
is an equation that is true.
When equations include unknown quantities, they may be true for only one value of the unknown:
is only true when n is 11.
If n = 10 then 2n – 5 = 17 is false.
They may be true for several values:
is true when n = 3 and when n = –3.
They may be true for all values of the unknown:
In formal mathematics you can use the sign ≡ to show that these expressions are ‘identically equal’:
Sometimes an equation is given as a definition or a formula for working something out. We can assume that someone else has done the work of showing it is always true. For example:
(definition).
(formula a learner could prove).
(formula that a learner could not prove!).
Activity 15 Always, sometimes or never true
Decide whether these equations are true for all values of the variable, or only some values or none.
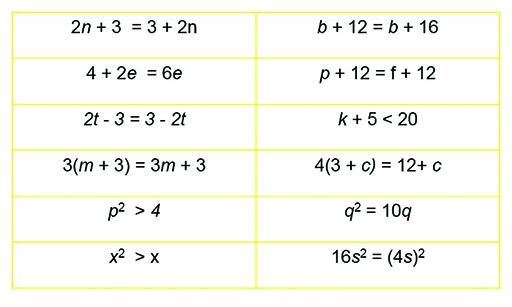
Discussion
Trying values will help you see that there are no values of b or m that make their equations true: they are never true. Using algebraic reasoning will help us see that e = 1, t = 1.5, c = 0, q = 10 are specific values that make their respective equations true. Also, some equations are true for a range of values: k sometimes true.
Finally the equations 2n+3 = 3+2n and 16s2 = (4s)2 are true for all values of n and s. The first is because addition is commutative, and the second because squaring applies to the whole bracket. They are always true.
