4.1 People and variation
Start by considering how you would begin designing an object that has to ‘fit’ to people, such as a simple desk or table. People using it will vary in size and these differences might be quite extreme (at the time of writing, the tallest person recorded had a height of 2.72 m and the shortest woman alive is 628 mm tall). What sizes should you start with? Is a single height acceptable? If not, how many sizes might you need?
Activity 6 Human variation
- a.What is the difference in height between the tallest recorded man (2.72 m) and the shortest living women (628 mm)? Calculate the answer in millimetres, then express this in metres to 1 d.p.
- b.What is the midpoint between the two extremes of height (the average of the two)? Would this be a sensible value to take as a starting point for a design?
Discussion
- a.The difference is found by subtracting the shortest from the tallest. Both heights need to use the same units before subtracting.
First, convert 2.72 m into mm (2.72 m × 1000 = 2720 mm)
Then subtract: 2720 mm − 628 mm = 2092 mm
So the difference in height is 2092 mm
Convert this to metres, by dividing by 1000: 2092 mm ÷ 1000 = 2.092 m
This is equal to 2.1 m, to 1 d.p.
- b.To find the midpoint you could halve the difference between the two heights and add it to the smaller height. Adding the two heights together and dividing by 2 will give the same result and is the usual way to find an average.
Average height = (2720 mm + 628 mm) ÷ 2 = 1674 mm, or 1.7 m to 1 d.p.
Without more information this would not be a sensible value to take as a ‘typical’ height. You need to know more about the distribution of heights, not just the extreme values, to make that judgement.
As it happens, 1.7 m is not too far from the average height of some groups of people, but you cannot be sure of this without more information.
Solving the problem of human variation is not a simple exercise. If you design a product to suit people at a particular height, you may then be limiting access for other people. Figure 13 shows a typical ticket machine in the UK. If you have used a machine like this, you might have wondered whether the designers took any account of human shape and sizes at all – or you might have found the machine comfortable to use. It all depends on your point of view.
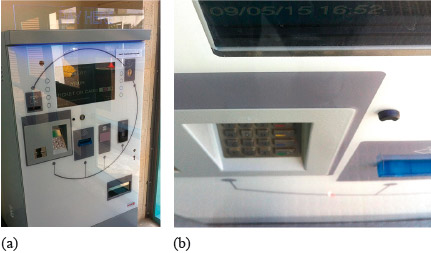
At some point decisions are required to be made about the properties of a design in progress. The ticket machine shown in Figure 13 had to be defined in terms of height, width and depth, and every component had to be located in relation to this to allow it to be manufactured. In making these decisions, however, you might solve the problem for some people but make it worse for others. Knowing how to think about difficult decisions like these can be useful as a designer. Because several different factors are involved, you need to use particular ways of thinking through this type of design problem.
