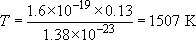2.2 Thermal effects in outline
Temperature is, of course, the measure of 'thermal' conditions. Nowadays it is measured by thermometers and expressed as a number on an agreed scale. Some features of thermometers and of their use are discussed in Thermometers and process control
The theoretical construct of temperature relates it to the kinetic energies of atoms. This gives clear insights into the way temperature affects the behaviour of materials. Energy is given to things to make them hot and taken from things to make them cold. Within solid matter, thermal energy is stored primarily in the kinetic energy of atoms. So, when we say that a particular object or substance has a certain temperature, we are in fact quantifying the amount of kinetic energy possessed by the atoms of that object or substance – see Box 1 Temperature and energy. To say that temperature is 'that which is measured by a thermometer' does not provide a definition which helps an understanding at the atomic scale.
Box 1 Thermometers and process control
Measuring temperature is not quite like measuring length with a ruler, where the length to be determined is compared with the length of the ruler. Thermometers convert the condition of temperature into other physical things, such as a resistance, voltage or pressure; we say they are temperature transducers. The temperature 'reading' is really a graduated indication of one of those other physical quantities. It is not very useful until the instrument has been calibrated against a defined numerical scale of temperature.
Here are some effects by which temperature is sensed and given number:
expansion of a liquid in a tube, often mercury in glass
resistance of a metal wire, platinum in good instruments
voltage output from a thermocouple, that is, the junction between wires of two different metals
pressure of a constant mass of gas kept at constant volume
brightness or colour of radiation emitted by a glowing furnace.
Industrial processes may require a steady or changing temperature. Changes may be spatial or temporal. Temperature may be tightly controlled by using a thermometer as the sensor in a feedback loop. On the other hand, if it is simply necessary to know whether the temperature is high enough for a process to work, or low enough for safety, the thermometer may merely monitor conditions or be coupled to an alarm to indicate when a threshold has been crossed. With such a diversity of uses, accuracy may not be the paramount virtue of a thermometer. Any of the following features may be of particular relevance for certain applications:
Accuracy: A thermometer will agree with the International Temperature Scale to a specified precision and will have been calibrated between some standard temperatures.
Range: The instrument must be able to give readings at the process temperature. For example, below −38 °C and above 360 °C a mercury-in-glass thermometer is useless. Radiation pyrometers are used to measure high temperatures.
Sensitivity: A process may need close control of temperature or need to be tolerant of some deviation. Close control needs a sensitive thermometer, that is one giving noticeable response to a small change in temperature.
Response time: A long response time means that short, sharp fluctuations of temperature are averaged out.
Repeatability: Actual values may be less important than that successive batches of a process get the same treatment.
Reliability: A malfunctioning instrument might close down the plant.
Corrosion-proofing: Furnace atmospheres can be very reactive chemically; the thermometer sensor may have to be protected by a ceramic sheath, which will slow its response.
Output: Telemetry of signals to a central control point favours thermometers with electrical output.
Compatibility: May need to be compatible with other instrumentation.
Cost: Cost per unit, or total cost of manufacturing, is almost always a consideration.
Exercise 1
In a mercury-in-glass thermometer, the length of a narrow column of mercury is measured. Explain how this is related to the local air temperature.
Answer
The mercury in a mercury-in-glass thermometer is presumed to be at the same temperature as the air that surrounds it. Changes in temperature lead to expansion of the mercury within the tube that encloses it – the tube is thick-walled, but the mercury can expand or contract along the length of the tube.
Box 2 Temperature and energys
How much thermal energy is associated with a given temperature? At this stage I just want to show you how to translate the absolute temperature of a substance, T in kelvin into the average energy, in joules, associated with the random motions of one of its particles. The link between energy and temperature will be discussed further in Section 4.
The conversion factor that links joules to kelvin is one of the basic physical constants that was established in the nineteenth century. Named in honour of one of the pioneers of the field, Boltzmann's constant has the symbol k and the value of 1.38 × 10−23 J K−1. Boltzmann's constant multiplied by absolute temperature (kT) gives the average kinetic energy of a particle in a substance that is at temperature T.
Consider room temperature, say 300 K. The average energy of particles in a substance at 300 K is:
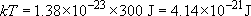
That's an inconveniently small number, and there are two ways to get something more useful from it.
The first is to consider a large number of particles, say a mole, instead of just one. In that case a mole of particles at room temperature consists of 6.023 × 1023 particles with an average energy of 4.14 × 10−21J.
A mole is a special quantity of a substance, measured in terms of the number of constituent particles (atoms or molecules), namely 6.023 × 1023 particles. One gram of hydrogen contains this number of atoms.
That means that at room temperature we can expect thermal energy to amount to
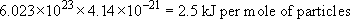
For comparison, you can put 2.5 kJ of energy into a small rechargeable battery, so I feel comfortable with that measure.
The other way to get a more meaningful description of thermal energy is to convert from joules to a unit more in tune with the energy of bonds between atoms. Such a unit is the electron volt (or eV) and you get it by dividing the value in joules by the electronic charge, e, another fundamental physical constant.
In terms of electron volts, 300 K works out as:
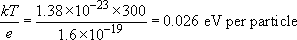
or about one-fortieth of an electron volt. Electron volts are convenient for measuring the energy binding individual atoms together – typically a few eV per pair of atoms – so at room temperature an atom in a solid with the average energy does not have enough energy to break free of its bonds.
You can build your confidence with this kind of conversion by doing the next exercise.
Exercise 2
Complete the following table that connects temperature with thermal energy in eV particle−1 and kJ mole−1.
| T/K | Average energy / eV particle−1 | Average energy / kJ mole−1 |
|---|---|---|
| 300 | 0.026 | 2.5 |
| 1000 | ||
| 1 | ||
| 300 |
Answer
| T/K | Average energy /eV particle−1 | Average energy / kJ mole−1 |
|---|---|---|
| 300 | 0.026 | 2.5 |
| 1000 | kT/e = 0.086 | 6.023 × 1023 × kT = 8.31 |
| 11600 | 1 | 6.023 × 1023 × kT = 96.4 |
| 36100 | kT/e = 3.11 | 300 |
SAQ 1
Calculate the temperature of a solid in which the atoms have an average energy of 0.13 eV.
Answer
Since 300 K corresponds with an average energy per atom of 0.026 eV, an average energy of 0.13 eV, some 5 times higher, will correspond with an absolute temperature that is also 5 times higher, namely 1500 K.
Alternatively, working from first principles,

Rearranging,