5.4 Critical modelling
Critical phenomena are the simplest to model of the three classes of temperature-dependent changes we have been examining. We don't need a power series such as 1 + αT+ βT2+…, nor exponentials such as exp(−Ea/kT). Instead we can describe the behaviour with logical expressions like these:
if T Tc, then property=subcritical value (or function)
if T > Tc, then property=supercritical value (or function).
For example, at atmospheric pressure,
if T 0 °C, then the 'natural' state of pure H2O will be ice.
It follows too that if T=Tc then both phases co-exist:
if T= 100 °C then pure H2O may exist as water or steam.
But it's not all ice, water and steam. Magnets, superconductors, aluminium alloys, steels and Box 12 Shape memory alloys are all solid engineering materials that make much use of critical phase changes. Are there effects here that could be used for making thermal switches for our electric water-boiler?
Box 12 Shape memory alloys
Components made from shape memory alloys (SMAs) have the ability to adopt a predetermined shape (one that is formed when its temperature is above some transformation temperature). Yet, when an SMA is 'cold', that is below its transformation temperature, it has a relatively low yield strength and can be plastically deformed into some other shape. On crossing above the transformation temperature again, the initial shape is recovered – you can imagine the excitement that must have accompanied the accidental discovery of this effect.
For instance, consider a helical spring formed from an SMA in its higher-temperature state (you can specify that to be anywhere in the range −100 to +100 °C). It will keep this shape even when cooler, though in the low-temperature state it will be considerably easier to stretch or squash. Suppose that, while cool, it is 'sprained' by pulling it out beyond its elastic limit so that when relaxed it is now twice the original length. On warming again past the transformation temperature it will begin to return to its original shape.
Can we do anything useful with it? Yes. If it is prevented from recovering the original form, stresses within the material will generate large forces on any external constraints. This phenomenon therefore provides a smart mechanism for remote actuation.
You should recognise that some structural phase change is behind this behaviour. We don't need a complete understanding of the mechanism on an atomic scale to make useful devices. Realising that phase changes are involved is enough, as it tells us to expect that we might be able to exercise some control over the transformation temperature through adjustments to chemical composition and microstructure.
The most common shape memory material is an alloy of almost equal parts nickel and titanium; it's called Nitinol. This particular alloy has very good electrical and mechanical properties, long fatigue life, and high corrosion resistance. The temperature at which the alloy 'remembers' its high-temperature form when heated can be adjusted by slight changes in alloy composition and through heat treatment. Above the transformation temperature, in the 'high' phase, the atoms are arranged in a pattern that metallurgists recognise by calling it face-centred cubic. In the 'low' phase the atoms are slightly displaced to take up a so-called body-centred tetragonal arrangement. In the process of rearrangement, no atoms move very far with respect to surrounding atoms, though as a whole large displacements of material can occur. Table 9 gives some typical physical properties for a nickel-titanium SMA.
| Property | Value |
|---|---|
| Density / 103 kg m−3 | 6.45 |
| Melting temperature / °C | 1240–1310 |
| Resistivity / μΩ m | 0.82 ('high' phase), |
| 0.76 ('low' phase) | |
| Ultimate tensile strength / MPa | 754–960 |
| Typical elongation to fracture / % | 15.5 |
| Typical yield strength / MPa | 560 ('high' phase), |
| 100 ('low' phase) | |
| Approximate elastic modulus / GPa | 75 ('high' phase), |
| 28 ('low' phase) | |
| Approximate Poisson's ratio | 0.3 |
As an actuator, Nitinol is capable of up to 5% strain recovery and can develop internal restoration stresses of around 300 MPa. If the transition temperature of an SMA is chosen such that room temperature is well below the transformation point of the material, then an electric current passed directly through the SMA is a handy way to initiate the recovery of its shape. The current generates enough heat to trigger the phase transformation. The SMA is heater, sensor and actuator – neat!
Example 3
Estimate the amount of force and distance of travel that could be triggered by warming a Nitinol wire 0.25 mm in radius and 20 mm long that had been 'permanently' stretched (5% strain) when 'cold'.
Solution
5% strain makes the 'cold' wire 1 mm longer. Using the figure of 300 MPa given for the level of stress driving the recovery of the original unstretched length, the force developed over the cross-section of the wire would be:
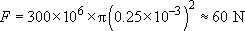
The recovery stress could lift a 6 kg mass a through a good fraction of 1 mm.
Shape memory alloys, however, are not appropriate for all actuator applications. You've got to take into account the forces, displacements, temperature conditions and cycle rates required in any particular situation. The advantages of Nitinol become more pronounced as the size of the application decreases. Large mechanisms may find solenoids, motors and electromagnets, or even explosive bolts, more appropriate. But in applications where such actuators cannot be used, shape memory alloys provide an excellent alternative.
SMAs also show superelastic behaviour when deformed at a temperature which is slightly above the transformation temperature. This effect is caused by stress-induced formation of the 'low' phase above its normal temperature. Because it has been formed above its normal temperature, the 'low' phase reverts immediately to an undeformed 'high' phase arrangement as soon as the stress is removed. This process provides a very springy, 'rubber-like' elasticity in these alloys with as much as 6% elastic strain beyond yield point.
Products containing SMAs have been around for many years, but we are often unaware of their presence because they are out of sight. One of the more visible applications is in 'indestructible' spectacle frames; these can be bent and twisted to a remarkable extent and then regain their original shape.