2.4 Period
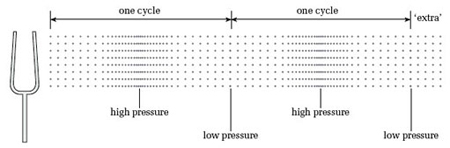
You saw in Section 2.3 that the prongs of the tuning fork vibrate cyclically. You also learned that a cycle of the prongs' vibration is a complete sequence of motion up to the point at which the motion starts to repeat itself. Another term for this repetitive kind of motion is periodic motion. The time taken for one cycle to occur is called the period of the vibration, and in theoretical work it is usually represented by T, or by the Greek letter tau, τ.
Properly speaking, in periodic or cyclical motion every cycle is identical to every other. With a practical tuning fork, however, no two cycles are identical. This is because each cycle is slightly weaker than the one before, as the vibration of the prongs diminishes from the moment the fork is struck. Nevertheless, it takes several seconds for a tuning fork to become silent, during which time there will be thousands of cycles of vibration. Thus over the course of a few cycles there will be very little change from one cycle to the next and we can regard the motion as periodic.
Activity 7 (Self-Assessment)
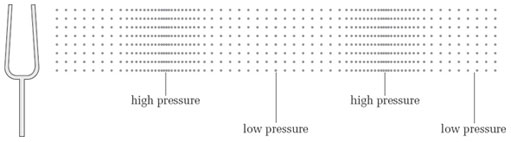
Approximately how many cycles of the fork's vibration were required to create the pressure pattern shown in Figure 5?
Answer
It took two complete cycles, and a little bit more, to create this pattern. To see why, notice that the right-hand fork is at the centre of the low-pressure part of the cycle, when the prongs are closest. To the right there are two other low-pressure zones, so to create this pattern two complete cycles were needed (Figure 5a), plus a bit extra to account for the region to the right of the diagram.
For a pressure wave created by a particular tuning fork, the distance from one high-pressure region to the next is fixed. A different tuning fork (that is, one that vibrates at a different rate) will produce a different separation of high- and low-pressure regions.