3.7 Looking at a standard: eye protectors

In many workplaces involved directly with manufacturing, there is the possibility of hazardous debris being flung around from various sources. Obvious examples are when components are being cut or otherwise machined, or if hot or toxic liquids are being handled.

A wide range of safety equipment is used in such environments. One of the most basic is for eye protection – that is, goggles or a visor to prevent any hazard from damaging the eyes of a worker (Figure 48).

Figure 48 Reconstruction of an accident in which polycarbonate lenses prevented serious damage to the wearer's eye

Show description|Hide description

Figure 48 is a side view photograph of a person wearing a pair of protective goggles. The left hand side of her face can be seen. A metal nail, approximately 60mm long, has struck the goggles and remains embedded in the lens. The pointed end of the nail has penetrated the lens and is very close to the wearer's eye. The nail must have been propelled towards the wearer at high speed and the lens has stopped it damaging the eye of the wearer.

Figure 48 Reconstruction of an accident in which polycarbonate lenses prevented serious damage to the wearer's eye

Eye protection equipment is sufficiently important to have a standard dedicated to it (designated BS EN 166: BS means it is a British Standard, and EN means it is also applicable across Europe). This means that anyone purchasing protective eyewear, whether for a school chemistry laboratory or a production workshop, can check its label to see if the product conforms to this standard and, if so, be confident that it will indeed be suitable for the job for which it is intended.

Before we begin to examine BS EN 166 further, it would be useful to review Writing decimal numbers and Precision.

The BS EN 166 standard is quite broad in its coverage of the requirement for a set of eye protectors. Eye protectors might have to be exposed to flying objects, small particles, splashes of liquid metal, and flashes of light bright enough to cause blindness. All of these hazards are encompassed by the standard, although it is not necessary for a particular product to be safe against every single one of them.

The most important part of the eyewear is the ocular, which forms the lens of the protective eyewear; that is, the clear barrier in front of the eye. The physical strength or endurance of the ocular is defined in the standard by a criterion, aptly named as robustness. The test for robustness in the standard is as follows:

The above extract states the minimum robustness. There is also an enhanced robustness criterion. If the ocular is removed from the spectacle frame for testing, this criterion is:

In both cases, the ball must not penetrate the lens, and the lens must not fracture or be greatly deformed if the protectors are to pass the test. If the ball passes through the lens or the lens breaks, then clearly the protectors would not be doing their job. If the lenses are tested whilst mounted in their frames, then they must not be pushed out of the frame, even if they remain intact when doing so. Figure 49 illustrates the tests.

Maximise

Figure 49 Ocular tests

Show description|Hide description

Figure 49 comprises 2 schematic illustrations of ocular tests. In each illustration a lens is represented by a flat circular shape of uniform thickness. The edge and top surface of the lens can be seen. In first illustration (labelled “a”) a ball is shown resting on the surface of the lens. The ball is labelled as having a “22 mm diameter”. Above the ball there is a vertical force arrow pointing down and touching the top surface of the ball. It is labelled “100N force on stationary ball”. In the second illustration (labelled “b”) the ball is shown in space above the lens. The ball is labelled as having a “22 mm diameter 43 g mass”. On the left side of the ball there is a vertical motion arrow pointing down. The arrow is labelled “speed of ball at impact = 5.1 ms ^-1 ”.

Figure 49 Ocular tests

From the introduction to this standard, and the answer to Activity 22, we can see that whilst any individual standard may appear self-contained, there is often subsidiary information that is needed by a manufacturer. BS EN 166 does not indicate how the measurements are to be performed, only what the requirements are. If the protective glasses are fitted with prescription lenses there are different standards that also need to be taken into account. In fact, the specifications for robustness given above specify that the test methods should be in accordance with the method given in another standard, BS EN 168. So, in addition to a standard on the requirements for protective lenses, there is also a standard that gives information on how the tests can be carried out for these requirements. One of the reasons for having standards is that they provide a way of disseminating advice and information on how to complete basic measurements, or use a particular product in a certain application.

Section 2: Normative references in BS EN 166 indicates other standards that are related to this one. Some of the related standards contain test methods (such as Personal eye protection – Non-optical test methods ); others cover eye protection for more specific use, such as for sun-glare filters. It soon becomes clear that no standard exists in isolation. The list of standards relating to eye protection is a good example. The standards for personal eye protection differ from those for spectacle lenses, and differ from those relating to protection from strong light sources – whether this is the sun or an artificial source of radiation (see Radiation, light and the visible spectrum ).

Radiation, light and the visible spectrum

In everyday speech, the word radiation is usually taken to mean the harmful emissions from radioactive material. However, in its correct scientific usage, radiation refers to various types of emission, only some of which are related to radioactivity, and only some of which are harmful. One of the commonest types of radiation is electromagnetic radiation, which is itself an entire spectrum of kinds of radiation, including for example light, microwaves, X-rays and some forms of damaging radioactivity. All varieties of electromagnetic radiation have the same fundamental nature, which is what the term 'electromagnetic' refers to. (Electromagnetic radiation is distinguished from particle radiation, which has a different nature.) We can use the example of light to illustrate properties that are common to all electromagnetic radiation.

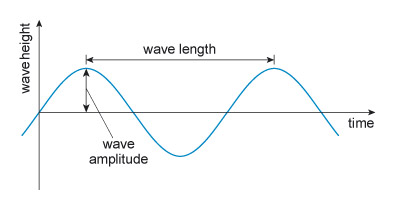
In simple terms, light can be envisaged as travelling as a wave, rather like the way the ripples in a pond travel out from their source. The distance between the peaks of the waves (Figure 50) is known as the wave length , or just wavelength.

Figure 50 Waves and wavelength

Show description|Hide description

Figure 50 is a mathematical graph. The horizontal axis is labelled “time” and the vertical axis is labelled “wave height”. Neither axis has measurement tick marks. The graph line looks like a sine wave. Following the graph line from left to right, it is first shown passing up through the origin rising at an angle of about 53 degrees. After that it starts to level out and briefly becomes horizontal forming a smooth peak some distance above the time axis. The line then proceeds to bend down until it is descending at an angle of about -53 degrees at which point it is crossing the time axis. The line then starts to level out again briefly becoming horizontal forming a smooth trough that is the same distance from but this time below the time axis. It then proceeds to bend up until it is ascending at an angle of about 53 degrees at which point it is crossing the time axis again. The line then starts to level out again briefly becoming horizontal forming a second smooth peak that is the same distance above the time axis. The line then proceeds to bend down until it is descending at an angle of about -53 degrees at which point it is crossing the time axis again. There is a vertical double ended arrow between the time axis and the first apex above the time axis. This arrow is labelled “wave amplitude”. There is a horizontal double headed arrow between the two apexes above the time axis. This arrow is labelled “wave length”.

Figure 50 Waves and wavelength

Maximise

Figure 51 The electromagnetic spectrum

Show description|Hide description

Figure 51 is a schematic illustration occupying the full page. It consists of 3 vertical sections. In the left hand section there is a vertical column 6 mm wide and 225 mm high. It is divided into 18 sections. On the left hand side of the column there is a vertical series of 17 numbers labelled “wavelength”. Each number is level with the end of a section in the column. Starting at the bottom and reading up, the wavelengths identified as “1 pm = 10 ^-12 m, 10 ^-11 m, 10 ^-10 m, 1 nm = 10 ^-9 m, 10 ^-8 m, 10 ^-7 m, 1 μm = 10 ^-6 m, 10 ^-5 m, 10 ^-4 m, 1 mm = 10 ^-3 m, 1 cm = 10 ^-2 m, 10 ^-1 m, 1 m = 10 ⁰ m, 10 m, 10 ² m, 1 km = 10 ³ m, and finally 10 ⁴ m”. As you read up the column, the wavelength is increasing. On the right hand side of the column there is a vertical series of numbers labelled “frequency”. Each number is level with a tick mark on the side of the column. However, these tick marks are at a point roughly midway through a section on the column. Starting at the bottom and reading up, the frequencies identified as “10 ²¹ Hz, 10 ²⁰ Hz, 10 ¹⁹ Hz, 10 ¹⁸ Hz, 10 ¹⁷ Hz, 10 ¹⁶ Hz, 10 ¹⁵ Hz, 10 ¹⁴ Hz, 10 ¹³ Hz, 10 ¹² Hz = 1 THz, 10 ¹¹ Hz, 10 ¹⁰ Hz, 10 ⁹ Hz = 1 GHz, 10 ⁸ Hz, 10 ⁷ Hz, 10 ⁶ Hz = 1 MHz, 10 ⁵ Hz and finally 10 ⁴ Hz. As you read up the column, the frequencies are reducing. The column is shaded light blue. However, the upper half of the 7 ^th section up the column is coloured like a rainbow between the labels “10 ¹⁵ Hz” (on the right) and “1μm = 10 ^-6 m” (on the left). This completes the description of the left hand section of the illustration. The middle section of the illustration consists of a vertical series of vertical double headed arrows being used to label different sections of the column. These arrows indicate ranges of wavelengths. Starting at the bottom, the first arrow, labelled “γ-rays”, comes from below the illustration (and so it has the lower arrowhead missing) and ends at 10 ^-11 m. The next arrow, labelled “X-rays”, is between 10 ^-12 m and 10 ^-9 m. It overlaps with the γ-rays arrow. The 3 ^rd arrow, labelled “ultraviolet”, is between 10 ^-9 m and a bit below 10 ^-6 m. The 4 ^th arrow, labelled “visible”, is between a bit below 10 ^-6 m and just below 10 ^-6 m. This range corresponds with the upper half of the 7 ^th section in the column that has the rainbow colouring. The 5 ^th arrow, labelled “infrared”, is between just below 10 ^-6 m and 10 ^-3 m. The 6 ^th arrow, labelled “microwave”, is between 10 ^-3 m and 10 ^-1 m. The 7 ^th arrow, labelled “radio etc.”, is between 10 ^-1 m and just above 10 ⁴ m. Along the radio arrow there is another series of labels which reads, starting at the bottom, “UHF (at about 10 ^-1 m), VHF (at about 1 m), SW (at about 10 m), MW (at about 10 ² m), LW (at about 10 ³ m) and VLF (at about 10 ⁴ m)”. They are not quite as evenly distributed as I have implied by the wavelengths. This completes the description of the middle section of the illustration. The right hand section of the illustration has 4 photographs that are being used to exemplify applications for (starting from the bottom) X-rays, infrared, microwave and UHF radio. The X-ray example is an X-ray radiograph of a human foot viewed from above and from the side. The bone structure is visible within the fleshy outline of the foot. The infrared example is an infrared image of what looks like a building. The image comprises areas of different colours that are being used to represent the intensity of radiation being emitted by the building. The darker areas emit lower levels (e.g. roof) and the lighter areas emit higher levels (e.g. the windows). The result is an image that lacks the detail of a photograph but the nature of the object can be recognised (i.e. a building). The microwave example is a picture of the Post Office Tower in London looking up from street level. The microwave radio aerials can be seen around the upper part of the tower. The UHF example is a photograph of an indoor aerial on top of a television set.

Figure 51 The electromagnetic spectrum

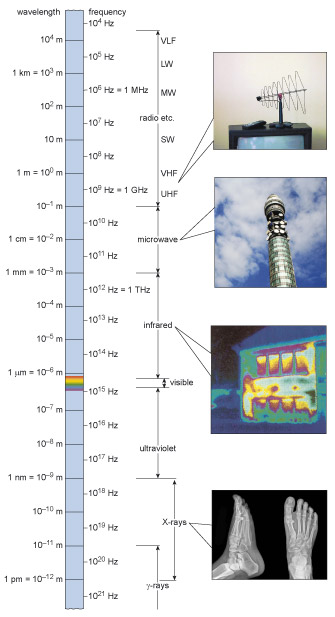
Wavelength can vary enormously, and as the wavelength changes so the properties of the radiation change. Since we classify various forms of electromagnetic radiation by their properties, the wavelength provides a useful way of specifying particular types of electromagnetic radiation. Figure 51 shows the electromagnetic spectrum. You can see that it ranges from gamma rays (one of the harmful types of radiation) at very short wavelength, through to radio waves at long wavelengths. Visible light comes somewhere in between. Here we see the use of a logarithmic scale for plotting data: the wavelengths shown on this figure range from 10 ^–13 m at the gamma-ray end (equivalent to a tenth of a thousandth of a billionth of a metre) to 10 ⁶ m (one thousand kilometres) at the radio wave end.

Another useful way of characterising electromagnetic radiation (or any other wave phenomenon, such as sound) is by its frequency. This is the number of waves that pass a stationary point each second, measured by counting the peaks per second. Although frequency is a simple concept – a number per second – it is given its own unit, the hertz, abbreviated to Hz. A frequency of 10 Hz is ten complete waves per second.

Let's now investigate the detailed procedure for the increased robustness test. BS EN 166 specifies that the test ball must strike the ocular with a certain speed: around 5.1 m s ^–1 (Figure 49). The standard also indicates that dropping the ball from a height of 1.3 m will provide this speed.

Of course the standard makes the assumption that the test is carried out on Earth, and that you haven't done something bizarre like cover the test rig in treacle! Gravity produces a force on everything at the Earth's surface, and when something is dropped this force causes the object to accelerate. You may also remember that the acceleration due to gravity is 9.8 m s ^–2.

Activity 25 shows it is possible to calculate how fast an object would be travelling after it had been accelerating for a certain length of time. We can also calculate how fast an object would be travelling after it had been accelerating over a certain distance.

If we use symbols to represent speeds, acceleration and distance, we can make this relationship easier to write. If there is an acceleration a over a distance s , then something that starts with a speed of u will end up with a speed of v. The mathematical derivation of the relationship between all these is outside the scope of this course, but the answer turns out to be:

• v² = u² + 2as

Thus if we know u , the starting speed of the object at a moment in time, we can work out what the final speed v will be after an accelerating or decelerating force acts upon it over the distance s.

In the case of a ball which is dropped, there is zero initial speed, so our equation becomes:

• v² = 2as

which, if we take square roots of each side, can also be written as:

The term v is the final speed after the object has moved through the distance s (fallen this distance in the case of an object being dropped). The term a represents the acceleration that it experiences. Properly speaking, a represents the magnitude of the acceleration. You will recall that acceleration is a vector, and therefore incorporates both a magnitude and a direction. The above equation takes no account of the direction of the acceleration.

Fortunately we have been able to use a bit of fundamental physics here to help us establish the speed of the ball – the fact that a ball dropped under Earth's gravity accelerates at a certain rate. If we had needed to measure the speed of the ball directly in order to prove that the test conditions were correct, it would have involved complicated and probably expensive equipment, all of which would have added to the final cost of the product. One of the key points that I want to convey here is that measurement methods are important. We have discussed already how the units of measurement are critical in ensuring compatibility of data. The way in which the measurements are made, in order to find values for specific quantities, is equally critical. This is why the eye protection standard has related standards concerned solely with the test methods.

You may have noticed, if you have looked at the standard, that impact resistance is not the only protection offered by eye protectors. They can also give protection against dust, and splashes of liquid metal, for example. Each of these elements of protection is tested in a particular way.

The consequence of all this is that as long as the conditions of the test, which are specified by the standard, are adhered to, the results for a particular make of eye protector should be the same no matter where the test is conducted. The fact that there is a standard for the test method as well as for the specific criteria for the protectors indicates the importance of the testing method. Without standardisation of this kind, it would be possible for manufacturers to claim that their product offered superior protection, whilst using non-rigorous testing methods to define their performance. As it is, eye protectors which conform to BS EN 166 will be labelled as having conformed to the relevant requirements.

This section has introduced you to the sorts of requirement that are aimed at in a standard for a particular product. The case we have examined, the standard for eye protectors, gives specific tests that the product must pass before it can claim to conform to the standard. This is typical of a standard for a product or a range of products. Such standards tend to define the function and performance of the product, and there may be tests the manufacturers must apply, or certain ranges of dimensions like length or weight, which the product must comply with.

This is just one type of standard. Examples of other types of standards are those dedicated to testing methods, and standards for processes or practices.