1.2 Quantitative evidence
1.2.1 What evidence are we reading?
Although we live in a society where a huge amount of information is available in the form of numbers, some of us still feel a mental fog descend when we are asked to deal with them. This is because numerical information is information in a very condensed and abstract form. A number on its own means very little. You have to learn to read it. Numeracy (the ability to work with numbers) is a skill that we can learn. It is a very useful skill, because it allows us to understand very quickly the scale of an issue or a problem.
Of course, even those of us with a fear of numbers use them all the time in our daily lives (for instance, to tell the time, to pay for our shopping, while watching a game of tennis, etc.), and some people develop quite remarkable numerical skill in particular settings. Experienced darts players often seem able to subtract, say, 47 from 73 almost instantly in their heads and will have thrown their next dart at Double 13 before most of us have had time to blink. This is a lot to do with practice, of course, but it is also to do with convention, knowing what the rules are. Most of us, asked to subtract 47 from 73 would, after a fairly mechanical process, produce the answer 26. For darts players, the subtraction is quicker because they've done it so often in the past, but, interestingly, it also results in a slightly different answer – Double 13, because of the rules of the game. What is the difference between 26 and Double 13 in darts? The difference between 26 and Double 13 may not appear much to someone unused to the conventions of darts, but it is absolutely crucial because it signifies one way of reaching 26 rather than the many others available. For experienced darts players, 26 and Double 13 are entirely different numbers, because you can score 26 in lots of ways, but Double 13 in only one.
Numbers come in many different forms, such as fractions, percentages, and averages. Most quantitative evidence is expressed as one or other of these basic forms of number, but it is important to recognise which is being used, to be able to convert one into another, and to recognise how they relate to each other. Return to the box below when in doubt.
Fractions are measures of proportion, like ‘a half’ (often written as ½) or ‘a quarter’ (¼) of any total.
Percentages are also measures of proportion, measuring the number out of a total of a hundred. So 65 per cent is 65 out of a hundred.
To express one number as a percentage of another when the total is more than or less than a hundred, divide the first number by the second and multiply by 100. So 92 as a percentage of 128 is ![]() , which is 71.875 per cent, or, roughly 72 per cent.
, which is 71.875 per cent, or, roughly 72 per cent.
To find the average of a group of numbers, add up all the numbers and divide the result by the total number of numbers. So the average of 6, 22, 35 and 29 is 92 (6 + 22 + 35 + 29) divided by 4, which is 23.
In social science, numbers are most often seen in the form of statistics, and these can be presented as tables, charts, diagrams or graphs, making them more readable.
Originally, ‘statistics’ meant the collecting of facts about the state. We now use the term to describe the techniques for working with large sets of numerical evidence and the presentation of summaries of their main features. For example, the bar chart is one of the most popular ways of presenting numbers. What, though, are the differences between this and some alternative representation of numbers, and why do we use one method rather than another? Let's take as an example some statistics on deaths in police custody, and ethnicity, taken from an article in The Runnymede Bulletin. There are four pieces of quantitative evidence in the article: the first is a simple line graph showing the increase in the number of deaths in police custody investigated by the Police Complaints Authority since 1995–96 (see Figure 4).
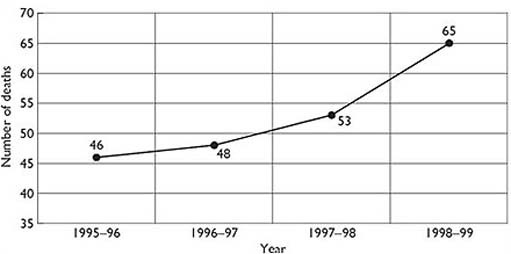
Line graphs are very useful for demonstrating the scale of change; this graph demonstrates a steady increase over the period covered (these statistics have been gathered since 1986, and to gain a bigger picture we would need to extend the time period covered). The graph doesn't tell us if the rate of increase has been the same for different ethnic groups (or for that matter different genders and ages), but it would be possible to show this using more lines on the graph if we had the statistics. (Statistics on the ethnic origin of people dying in police custody have been gathered only since 1996.)
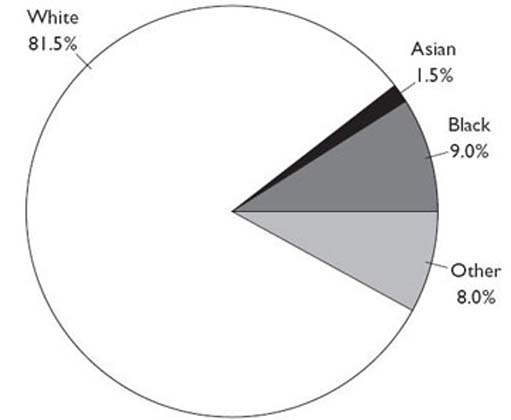
The relationship between deaths in police custody and ethnicity is explored in the article using three pieces of evidence. First, the ethnic origin of those recorded as dying in police custody in 1998–99 is shown in a pie chart (see Figure 5).
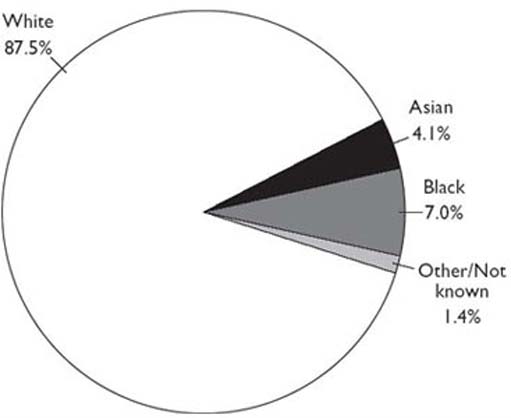
This gives us a pretty clear picture of the proportion of different ethnic groups dying in police custody. Pie charts are a good way of demonstrating the relative size of a small number of categories. Next, another pie chart shows total arrests by ethnicity in 1997–98 (see Figure 6).
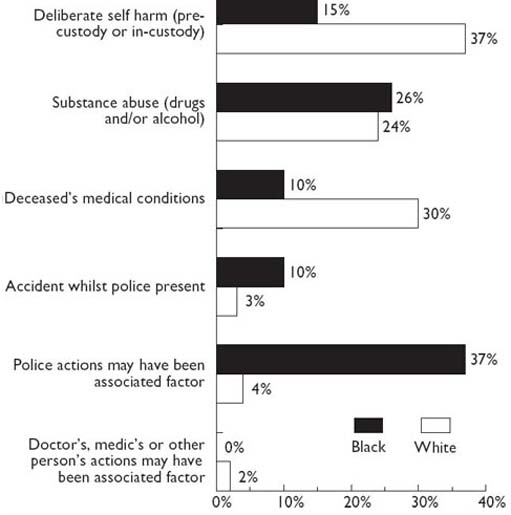
Again, this clearly demonstrates the proportion of people of different ethnic groups involved. A more complex picture is presented in the next piece of evidence, a bar chart where ethnicity and cause of death are linked together (see Figure 7).
This clearly shows that there is a marked difference in cause of death between two of the ethnic groups covered in the previous figures. Bar charts are good at making relatively complex information accessible in a single ‘picture’.
Warning: the ‘facts’ never speak for themselves
However quantitative evidence is presented, it always needs interpretation. A good example of this is the last piece of evidence in Figure 7, where the evidence shows that a much higher percentage of black people than white die in custody as a result of ‘police actions’ and ‘accidents whilst police are present’. This could be explained in radically different ways. Some might argue that the statistics are the result of black people being inherently more aggressive and difficult to arrest, hence requiring more ‘physical’ policing resulting in higher risk of fatality or injury. Others might argue that the statistics are a result of racism. To decide between the two we need all of the elements on the circuit of knowledge, not just the evidence.
