1.2.6 Stage 4: Extracting the information
When you are absolutely sure that you know what the diagram or table is all about, start to look for patterns, for discrepancies, for peaks and troughs, for anything unusual. Diagrams and tables are highly patterned information, and they often tell a relatively simple story underneath. Don't get bogged down in the relationship between individual numbers, but look to see whether one relationship is like another, or whether one set of numbers stands out significantly from the rest.
Warning: statistics can lie
One of the main advantages of using numbers and diagrams as evidence is that they can give us a very clear idea of the scale of social issues, and they can give us a lot of information very quickly. However, they can also mislead us, usually accidentally, but occasionally with the full knowledge of those who produce them.
Let's take an example of a small village in a poor country where 100 workers earn just £100 each per year, 10 managers earn £1,000 per year, and the major landowner earns £10,000 per year. The total income of the village is therefore £30,000. Now, suppose we were asked to provide evidence of the income distribution in the village, and for reasons of our own we wanted to paint a rosy picture. We could say that the average income of this village is £270 (£30,000 divided by 111, the number of earners). This might make people believe that most inhabitants of the village earn around about this figure, but as we know it is almost three times as much as most villagers' income, so it doesn't really give a fair picture. Alternatively, we could give the median income, which is the income at the point where 50 per cent of the people are above it, or the mode, which is the most common income. Both of these are £100. But this ignores the fact that some people earn a great deal more. Does this give a fair picture? To give a fairer picture of income distribution in the village, we could give the upper and lower deciles (the average income of the lowest-earning 10 per cent and highest-earning 10 per cent of the villagers), which would be £100 (£1,100 divided by 11) and £1818 (£20,000 divided by 11), but this would still miss the fact that one person earns a lot more than everybody else. We need to be on our guard when we read numbers. They may only tell a partial story. (A similar example appears in Sardar et al., 1999, p.52.)
Activity 2
Let's try looking at an example of reading statistical evidence. There has been a great deal of discussion in recent years about changes in our patterns of travel, because of concern about car use and its impact upon the environment.
What does the table reproduced below tell us about car use?
Can you detect any patterns or trends over time?
| Miles per person per year | |||||||
|---|---|---|---|---|---|---|---|
| 1975/76 | 1985/86 | 1989/91 | 1992/94 | 1995/97 | 1998/00 | Percentage change from 1989/91 to 1998/00 | |
| Walking (including short walks) | 255 | 244 | 237 | 199 | 195 | 186 | −21 |
| Bicycle | 51 | 44 | 41 | 38 | 39 | 38 | −8 |
| Private hire bus | 150 | 131 | 123 | 110 | 105 | 109 | −12 |
| Car | 3,199 | 3,796 | 4,806 | 4,954 | 5,187 | 5,355 | 11 |
| Motorcycle/moped | 47 | 51 | 37 | 32 | 30 | 30 | −19 |
| Van/lorry | 183 | 228 | 301 | 281 | 262 | 218 | −28 |
| Other private (inc. invalid carriages, Dormobiles, etc.) | 16 | 33 | 34 | 43 | 40 | 26 | −21 |
| Buses in London | 57 | 39 | 36 | 40 | 50 | 47 | 30 |
| Other local bus | 372 | 258 | 238 | 219 | 202 | 199 | −17 |
| Non-local bus | 54 | 109 | 124 | 96 | 93 | 100 | −19 |
| LT underground | 36 | 44 | 49 | 50 | 51 | 57 | 16 |
| Surface rail | 289 | 292 | 366 | 298 | 294 | 371 | 1 |
| Taxi/minicab | 13 | 27 | 42 | 38 | 43 | 62 | 47 |
| Other public (including air, ferries, light rail, etc.) | 18 | 22 | 40 | 41 | 75 | 45 | 13 |
| All modes | 4,740 | 5,317 | 6,475 | 6,439 | 6,666 | 6,843 | 6 |
| Percentage of mileage accounted for by car (inc. van/lorry) | 71 | 76 | 79 | 81 | 82 | 81 | — |
| Average trip length | 5.1 | 5.2 | 5.9 | 6.1 | 6.3 | 6.6 | 12 |
All figures have been rounded to the nearest whole number. This gives rise to rounding differences in the percentage change and total figures.
Discussion
We noted that the number of miles travelled in a car per year is steadily rising (11% increase from 1989/91 to 1998/00). Car use is one of the few modes of transport (other than London bus and underground) to show a significant increase over the period. Taxi and mini cab travel has risen sharply over the whole period. The percentage of mileage accounted for by private car travel has risen consistently to around 81% of all travel and average trip length has risen consistently and grown by 12% from 1989/91 to 1998/00. Such data do not tell us the reasons, nor the outcomes but they do offer evidence of trends in patterns of transport use.
Gender differences in educational achievement: working with numbers
Social scientists have constructed gender categories and have examined in particular how children learn to use these categories. The question of how far these gendered identities influence children's behaviour in schools and, specifically, their achievement in examinations is one that is posed during such an examination. Until recently the dominant political concerns in this field were about girls' under-achievement in school. Recently the issue of boys' under-achievement has hit the headlines and boys' GCSE results have helped trigger these concerns.
Figure 8 below is a bar chart which shows the GCSE results of girls and boys in different subjects. Bar charts are a very useful way to display information, so let's pause here a moment and work through the skills involved in reading this one.
The bar chart shows results in percentages so there are two skills involved. One is understanding percentages, the other is reading bar charts displaying them. Let us start with percentages.
Percentages
Percentages are a very common and useful way to describe exact parts of something. The most common way to describe a part of something is to use forms like ‘a half’ or ‘a quarter’, but when you want to describe a part which is not a half, a quarter or a third, or you want to be very precise, then percentages are very convenient. They are used frequently in newspapers – and in social science courses.
This section of the course aims to ensure you really understand this useful idea. If you are sure you do, proceed to the activities below to check, but for many people percentages are a bit of a mystery; so here is a short introduction.
You recognise a percentage by the symbol %. It is read as ‘per cent’ and it means ‘out of a hundred’. So, if out of 100 children in a room, 5 are girls and 95 are boys you can say that 5% of those in the room are girls and 95% are boys.
Things are not quite so straightforward if there are fewer or more than 100 children in the room. Then, in order to work out the percentages of girls and boys, we need to do some calculations. The calculations are worth doing because in the social sciences they offer an easy way to compare proportions when you need to do so. Let us see how this works.
Activity 3
Suppose there are only 50 children in the room. 5 of them are girls and 45 are boys.
What percentage of the children are girls? Try to work it out for yourself first. If you do not know how, just read on.
Discussion
10% of the children are girls. Here are a couple of ways of working that answer out. See which is clearer to you.
Out of 50 children, 5 are girls. To calculate the girls as a percentage of the total, we have to treat the whole group of 50 as if they were 100. We need to multiply by 2 to turn 50 into 100. Then we multiply the number of girls by 2 as well. 5 out of 50 is the same as 10 out of 100 or 10%.
Alternatively, we can state the proportion of girls as
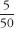
Then to turn the fraction into a percentage, you just multiply by 100
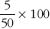
You can work out the answer to that by hand or on a calculator. We advise a calculator! On a calculator, you first divide 5 by 50, then multiply the answer by 100. Try it now, and check that you get the answer 10.
By hand, you need to ‘cancel’ the fraction like this: first divide top and bottom of the first fraction by 5

then multiply the result by 100
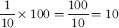
You do not need to understand that calculation to work happily with percentages. The calculator method is just fine.
We can now turn that calculation into a rule:
Rule 1: Calculating a percentage. To express one number as a percentage of another, divide the first number by the second and multiply by 100.
Activity 4
Check your understanding of this rule by using a calculator to answer the following:
-
You get 42 marks out of 70 for an assignment. What is your mark as a percentage?
-
In an (imaginary) UK opinion poll in 1999, 2,500 people were asked who Gordon Brown was. 425 did not know. What percentage had never heard of the Chancellor of the Exchequer?
-
In a group of 325 people, 65 own a computer and 52 have access to one at work. Express that statement in percentages.
Discussion
-
60%
-
17%
-
20% own a computer and 16% have access to one at work.
Reading bar charts
Now let us return to the bar chart and have a careful look at this way of displaying information. Bar charts are really good at showing quantitative data in ways which allow the reader to absorb and understand them. They are worth a bit of effort to get used to them. We are not going to worry here about drawing them, just reading them.
Figure 8 represents the results for English and Maths, for girls and for boys. Start by reading the title: it says these are results for 16-year-olds showing the percentage of girls and the percentage of boys achieving GCSE results A*–C.

Now look at the axes, that is, the horizontal line across the bottom and the vertical line up the left-hand side. Read the labels on these axes carefully. The vertical one shows the percentages defined in the title, from 0 up to 60%. The horizontal axis reads ‘92’, ‘93’ … ‘97’, so this shows a series of years. The chart is going to show percentages for each year, so we can look at changes over time. Finally there is a key or legend: this tells us which of the bars for each year refers to girls, and which to boys.
Murphy and Elwood: constructing a social science argument
Having identified some key differences in boys' and girls' GCSE results in the 1990s, what are possible explanations? To answer this question, researchers look at biological and cognitive factors and a range of social factors. They focus on the arguments of Murphy and Elwood: that children's gendered identities lead them, in general, to tackle school-work differently and to perform differently in exams. Murphy and Elwood aim to provide an explanation of the different performances of girls and boys by building up an argument about the links between gendered identities and school performance. We now want you to use Murphy and Elwood's argument to help you think further about how social science arguments are constructed.
Three claims form the core of Murphy and Elwood's work, listed below.
-
Claim 1: Boys and girls foster different interests, attitudes, and behaviours prior to attending school, which are then perpetuated within school.
-
Claim 2: Feminine and masculine identities are perceived in particular ways by teachers, with consequences that may impinge on achievement.
-
Claim 3: In their school-work, girls and boys draw upon the different interests and skills that they have developed through their gendered experiences.
How are these claims put together? How is the argument constructed? Look back at your notes and think about what is involved in the first claim.
Claim 1
This claim draws upon biological, cognitive and social arguments. The interaction between these different components in early childhood development are seen as maintained in the school context. What constitutes typically gendered behaviour draws upon stereotypical gendered characteristics.
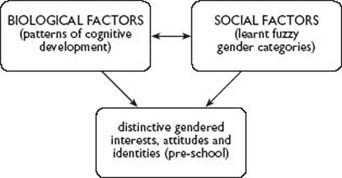
Claim 2
This claim argues that those pre-school attitudes and identities are reinforced by teachers, and lead to distinct gendered patterns of behaviour in skills with direct consequences for school performance and achievement.
Claim 3
This claim focuses on the cognitive skills which girls and boys have developed through their early childhood development. These skills directly impact on performance, though in different ways in different subjects.
The three claims combine to produce an argument which seeks to explain gendered behaviour and differential educational achievement in terms of an interaction between individual girls and boys and the social world which they inhabit. Typical gendered attitudes and attributes are reinforced and rewarded in the school setting so that children and young people develop and reinforce particular skills. Cognitive skills are developed and reinforced through social and educational experience. What is typical draws on gendered categories. There is scope for reconstruction of categories here, and not everyone conforms, but the process is cumulative and interactive. It develops to include more factors and shows how gender identities are reinforced through an interaction between different interrelated factors, biological and social.
Thus the argument is both developed and expanded to include a range of evidence which supports the claims that are being made. The argument uses this evidence to show that biological factors, such as patterns of cognitive development, are closely linked to social factors, such as learned gender categories. These cognitive skills are developed both pre-school and subsequently at school, supported by the responses of teachers to children's pre-school development, creating a reinforcement of patterns of gendered performance.
Activity 5
Look first at the English GCSE part of the bar chart. Explain in words what it shows.
Discussion
It shows that girls have consistently done better than boys, and that this has not changed a great deal. Around 60% of girls achieved A*–C grades each year from 1993 to 1997, and a slightly lower percentage in 1992. Only about 40% of boys managed the same grades in 1992 and from 1995 to 1997, and somewhat more than 40% in 1993 and 1994. There is no trend: that is, the percentages are not consistently rising or falling over the six years.
Activity 6
Now Maths: what does it show, and how does it compare with English?
Discussion
In Maths, the performance of boys and girls is very similar. And there is a trend: results have been getting somewhat better over the six years. Comparing Maths and English, we see that boys produce very similar results in the two subjects – hovering around 40% obtaining A*–C grades in both. However, a higher percentage of girls do well in English than in Maths.
