Skip to main content
Printable page generated Thursday, 25 April 2024, 4:15 PM
Use 'Print preview' to check the number of pages and printer settings.
Print functionality varies between browsers.
Unless otherwise stated, copyright © 2024 The Open University, all rights reserved.
Printable page generated Thursday, 25 April 2024, 4:15 PM
 TI-AIE: Creating contexts for abstract mathematics: equations

TI-AIE: Creating contexts for abstract mathematics: equations

What this unit is about

Constructing, writing, reading and solving equations play an important role – not only in the school curriculum but also in the process of mathematical thinking. Equations are important in other subjects as well, such as science, business and commerce. In school mathematics, the focus tends to be on solving equations, and students often see this as a mechanistic procedure without really understanding what equations are about. Thinking about equations can include the equation’s properties, how they come about and what they represent.

This unit will address some of these ignored aspects by suggesting ways in which you can help your students in understanding equations by making links with graphical representations of algebraic equations and expressions, and by juxtaposing differences and sameness. The activities are also designed to elicit misconceptions and offer ways to discuss these. Concept and mind maps are used to help further with students’ understanding of these mathematical concepts.

What you can learn in this unit

  • How to work with your students on visualising and contextualising equations.
  • Some ideas on how to use contexts to help your students to see the purpose in equations.
  • How to use concept maps and mind maps to build an understanding of the mathematical concept of equations.

This unit links to the teaching requirements of the NCF (2005) and NCFTE (2009) outlined in Resource 1.

Specialised mathematical vocabulary used in this unit

An algebraic equation is of the form p(x) = q(x):

  • ‘p(x)’ and ‘q(x)’ are algebraic expressions.
  • ‘p(x)’ is the expression on the left-hand side (LHS) of the equation in this example.
  • ‘q(x)’ is the expression on the right-hand side (RHS) of the equation.
  • ‘=’ indicates that the LHS is equivalent to the RHS.
  • A solution set is ‘s’, the set of values that satisfy a given set of equations or inequalities. The sign of equality in such an equation means that one side results in the same value as the other for the solution set ‘s’.

Pause for thought

  • Thinking about your own classroom, what are the common issues your students are struggling with when working on equations?
  • Thinking back to your experiences as a mathematics student, what were the common issues you struggled with when working on equations?

1 Thinking about the rules for solving equations

To find a possible value (or values) of x, where p(x) is the same as q(x), we need to ‘solve’ the equation p(x) = q(x).

There are certain rules that are sometimes helpful to solve equations. Those rules are often memorised by students – or partially and incorrectly memorised, and many mistakes are made in the process of applying these rules. Part 1 of Activity 1 is intended to make students think about where these rules come from, and why and when they can be used. Part 2 aims to make the students aware of different types of equations. Students’ learn more effectively if they are able to talk to other students about their thinking. Asking them to ‘discuss this with your classmate’ is a good strategy to use.

Before attempting to use the activities in this unit with your students, it would be a good idea to complete all (or at least part) of the activities yourself. It would be even better if you could try them out with a colleague, as that will help you when you reflect on the experience. Trying the activities yourself will mean that you get insights into learners’ experiences that can in turn influence your teaching and your experiences as a teacher. When you are ready, use the activities with your students. After the lesson, think about the way that the activity went and the learning that happened. This will help you to develop a more learner-focused teaching environment.

Activity 1: learning about equations

Part 1: Rules of the solving equations game

Tell your students the following:

When solving equations there are certain rules, or truths, that can be useful. You will probably know these already. The aim of this activity is for you to say where these rules come from, why and when they can be used. To help you make sense of these general statements it is useful to think of examples that fit these general statements.

Which of the following rules can always, sometimes or never be used to solve equations? How do you know?

  • If p(x) = q(x), then p(x) + c = q(x) + c.
  • If p(x) = q(x), then p(x) – c = q(x) – c.
  • If p(x) = q(x), then p(x) .c = q(x) .c.
  • If p(x) = q(x), then p(x)/c = q(x)/c.
  • If [p(x)] 2 = [q(x)]2, then p(x) = – q(x).
  • If [p(x)] 2 = [q(x)] 2, then p(x) = q(x).

Create a graph of each of these rules and compare them with the original p(x) = q(x). Discuss with your classmate what has changed in these graphs and what stays the same.

Part 2: None, one and infinite solutions

Tell your students the following:

Compare the answers for the three equations below. How are your results different? Why are they different?

  • Find x, if 4(x – 8) = 4x – 32.
  • Find x, if 4(x – 8) = 4x – 30.
  • Find x, if 4(x – 8) = x – 32.

Plot the LHS and RHS of each equation on the same graph and interpret your algebraic results graphically. What do you notice?

Consider the equation 2x – 3y = 8. Create a graph of this equation. Now create a graph of another equation of the form ax + by = c, so that the two equations have:

  • the same set of solutions
  • no common solution
  • only one common solution.

Consider again the equation 2x – 3y = 8. Now write another equation of the form ax + by = c, so that the two equations have:

  • the same set of solutions
  • no common solution
  • only one common solution.

During this activity, encourage your students to talk to each other about their ideas and to help each other.

Case Study 1: Mrs Rawool reflects on using Activity 1

This is the account of a teacher who tried Activity 1 with her secondary students.

It was a good idea to have done Part 1 before embarking on the next one. The students at first found it hard in Part 1 to make sense of the general statements. The hint of using examples to understand what the statement was saying worked well.

I walked around the classroom observing how the students tackled Part 2 of the activity. I noticed they had several misconceptions. I thought about how I would deal with this. I took a decision: I asked them all to give their solutions and wrote all of them on the blackboard, whether they were correct or incorrect. I then asked, ‘How do you know this is the correct answer? Discuss it with a classmate.’ Most of the mistakes related to manipulation of the equation. Because we had just done Part 1, this could now be discussed. I noticed, though, that although the students might now know why the ‘rules’ of manipulation are what they are, they needed some practice to recognise these in the setting of solving equations. The other misconception, and this surprised me, was that several students did not know the role of brackets in 4(x – 8). They simply removed the bracket and wrote 4x – 8. So we had a discussion as to why a bracket is given and what it implies.

Creating graphs really helped to make sense of the equations. Renu was the first to finish, so I asked her to help some of the others who were struggling to get a solution. It actually surprised them that the graphs looked different. They became much more confident in their discussions about what was the same and what was different in the three equations.

Video: Using questioning to promote thinking

You may also want to have a look at the key resources ‘Using questioning to promote thinking’ and ‘Talk for learning’.

Reflecting on your teaching practice

When you do such an exercise with your class, reflect afterwards on what went well and what went less well. Consider the questions that led to the students being interested and being able to get on and those where you needed to clarify. Such reflection always helps with finding a ‘script’ that helps you engage the students to find mathematics interesting and enjoyable. If they do not understand and cannot do something, they are less likely to become involved. Use this reflective exercise every time you undertake the activities, noting, as Mrs Rawool did, some quite small things which made a difference.

Pause for thought

Good questions to trigger this reflection are:

  • How did this activity go with your class?
  • What responses from students were unexpected? Why?
  • What questions did you use to probe your students’ understanding?
  • Did all your students participate in the activity? Were there any students who were less confident about the graphs? How will you support their learning in the next lesson?
  • What points did you feel you had to reinforce at the end of the lesson?

If you have access to the internet, there are some mathematics graphing software packages available such as Autograph. They can help your students to visualise the graphs of equations.

2 Contextualising equations

As discussed earlier, equations are thought of as purely symbolic expressions for most of the time. The idea is almost always to find a solution of the equation through symbolic manipulation. Each manipulation, if done in accordance with conventional rules, leads to an equivalent equation that is simpler (easier to solve) than the previous one. More meaning can be given to the algebraic notations of equations by linking them with their graphical representation, as in Activity 1.

This however is still a symbolic approach to seeing and solving equations. It does not address the concept that each equation can be seen as a representation, or model, of a real-life situation.

You can think up a story for each equation to explain it. Working on developing such stories helps to:

  • enliven mathematics
  • allow students to think about the mathematical processes involved in making sense of a situation
  • make the students actually think about the distinction between variables and constants, and how that relationship can be changed if they decide to change your assumptions.

The next activity offers a gradual introduction to thinking of stories to contextualise equations. This will prepare your students for more complex problems later in their mathematical learning.

Activity 2: Thinking of contexts

Part 1: Changing a story

Tell your students the following:

Imagine if Mohan participated in a quiz show where he won a certain amount of money for every question that he answered correctly, with the rule that for each correct answer he gets twice the amount he got for the previous question. If, before attempting the fifth question he had already won Rs. 30,000, the equation 15x = 30,000 can be formed, where x is the amount he won to answer the first question correctly.

Can you find another context – another story – that is represented by the equation 15x = 30,000?

Part 2: Thinking of a story

Preparation

It is not important whether the students’ answers are right or wrong. Focus on (and encourage) your students’ ability to come up with a creative and imaginative context to embed the mathematics and then share how they figured out the context. A good trigger word is ‘Imagine …’

Write the equation 2x + 5 = 12 on the blackboard.

The activity

Tell your students to use their imagination to write a word problem that contextualises this equation. Ask them to share their ideas with the rest of the classroom.

Part 3: Creating equations and then thinking of a story

Preparation

This is a continuation of Parts 1 and 2, which focused on contextualising word problems. You will now ask your students to first create equations and then think of word problems that fit these equations.

Write Table 1 on the blackboard:

Table 1 A collection of expressions.
22910.503/5
x3x2.5x5x/6
5x – 834x – 12 5.5x + 1.7(2/3)x – 4/5
x22x2x2 + 1 x2 – 2

The activity

First create an equation from Table 1 by selecting a collection of expressions with the following rules:

  • Two or more expressions chosen from the same row are always added and must lie on the same side of the ‘=’ sign.
  • Expressions from different rows should be on different sides of the ‘=’ sign.

Then frame a word problem that describes the equation.

For example, if you chose 2 and 10.50 from the first row, and 3x and 2.5x from the second, you get:

3x + 2.5x = 2 + 10.50

        5.5x = 12.50.

A sample word problem might be: ‘The area of a rectangle of length 5.5 cm and width x cm is 12.5 cm2.’

If you have access to large pieces of paper ask your students to write their problems on this paper and display it around your classroom. Ask students to walk round and read each other’s word problems. You could ask them to identify word problems that they like, copy them down and check them, giving feedback to the students who wrote them.

Video: Storytelling, songs, role play and drama

For more information read Resource 2, ‘Storytelling’.

Case Study 2: Mrs Mohanty reflects on using Activity 2

There was a lot of hesitation initially in doing Parts 1 and 2, as the students had never made up such kinds of questions. They needed some prompting and reassurance that any kind of story that would fit was fine; that it did not need to be plausible, or that it might help to start their story with ‘Imagine …’. For the equation 15x = 30,000, Meena said that if 15 things were bought and the total price paid was Rs. 30,000, then x would be the price of each. Sharad suggested that x could be the number of days worked and Rs. 30,000 the money earned.

Now I told them to try the one that was given in Part 2 – that is, 2x + 5 = 12. There were no takers. I decided to simply wait, without saying anything. After about 90 seconds, which seemed like a lifetime but was actually not that long, Rohit very hesitantly said if he was going to travel by autorickshaw and Rs. 5 was the minimum amount for the first kilometre and Rs. 2 for every subsequent kilometre, then x km would be the distance he had travelled. Meena at once said no, you would have travelled x +1 km. Anju came up with the example that there were two groups of children on the playground and then five more children arrived and there were now 12 children in total – how many had been in the groups? Because the answer would be 3.5 children, a discussion followed about the difference between natural numbers and non-rational numbers, which was interesting.

The discussion of Part 3 was mainly that they could form an equation easily but could not always find a realistic enough situation to describe all of them.

Like Mrs Mohanty your students may find these activities unfamiliar and need practice to become confident at thinking to word problems. Try to use this technique in more maths topics, as it will help your students to make sense of the mathematics equations.

Pause for thought

  • What questions did you use to probe your students’ understanding?
  • Did you feel you had to intervene at any point? What points did you feel you had to reinforce?
  • Did you modify the task in any way? If so, what was your reasoning for this?

3 Using pictures to make students think about related variables

Equations relate variables and constants. Apart from identifying which is which, another interesting question in mathematics is to what extent variables in a given context relate to each other. A good way to help students understand this is by asking them to look at pictures and come up with their own ideas of related variables.

In the next activity, students are presented with four situations. They need to identify all the variables they can think of and then determine the pairs that may be related.

Activity 3: Finding related variables

Preparation

For this exercise, divide the class into small groups (pairs, threes, or fours) and ask them to work collaboratively. Decide how you will organise the groups. It is important to do this before the lesson to save time during the lesson. Use the images from Figure 1 below or use your own similar images, for example from newspapers.

The activity

Tell your students to look at the four images in Figure 1. In each case, make a list of variables that may depict any aspect relating to the image.

Figure 1 Four photos: what are the related variables?

For each list, prepare pairs of variables that you think may be related. Create a specific context involving these variables and represent that context as an equation. Use your imagination!

At the end of the activity, ask groups to share their thoughts and then evaluate them.

Case Study 3: Mr Bhatia reflects on using Activity 3

I decided that the work would be done in groups of fours. We made 11 groups, and pictures were assigned to each group with the instruction to work out the different variables and produce equations.

They all came up very enthusiastically with the different variables in the pictures. Without further prompting they could also, to some extent, identify the ones that they thought were related and those they thought were not. Then some groups seemed to get stuck. They could not think of how to form connections.

I had been circulating around the class but I paused the activity at this point and asked for some examples. I said even a tiny idea would help all others to get unstuck.

Shobha and her group were working on the picture of the lady frying something and said that if there were ‘y’ people in the house and each ate ‘x’ puris, then if the lady made 40 puris, xy = 40. Mona from the second group was working on the same picture and came up with a context related to the material used. They decided that the lady was making pakoras. So if she used ‘x’ kg of besan (gram flour) and ‘y’ kg of potatoes, and if ‘a’ was the cost per kg of besan and ‘b’ was the cost per kg of potatoes, her expense would be ax + by.

I praised these two volunteers and saw that their examples were enough to move the other groups on in their discussions. After a while I asked each group to make a presentation of one of their ideas and we had some good presentations. I was actually very impressed with how easily and naturally the students introduced several variables within an equation – something I would have been reluctant to expose them to from the textbook.

Pause for thought

  • What questions did you use to probe your students’ understanding?
  • What responses from students were unexpected? Why?
  • Did you feel you had to intervene at any point? What points did you feel you had to reinforce?
  • How did you evaluate your students’ learning in this activity?

4 Concept maps and mind maps

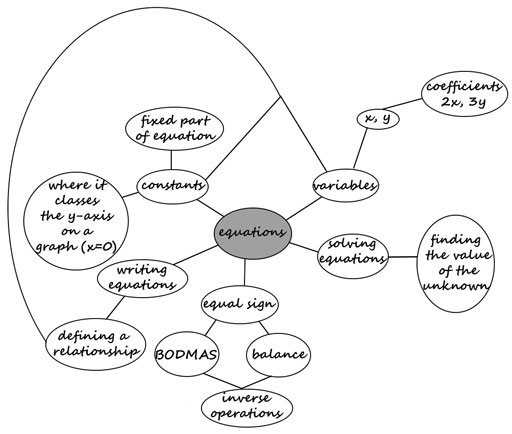
A good tool to help students see mathematical connections is a concept map. A concept map can be seen as a representation of a person’s knowledge about relationships between concepts relating to a specific topic (Novak and Gowin, 1984). Typically, it is a series of words or phrases to represent the concept (as a node), and a line joining it (a link) to another concept to express the relationship between the two. This is a good tool and strategy to help students explore and review their own understanding. It can also be used as an assessment tool to find out what the students know and what their misconceptions are. A mind map is similar to a concept map, but mind maps have a centre whereas concept maps can be linear. There are lots of examples and information on concept and mind maps on the internet – an example of a mind map is in Resource 3.

The next activity asks the students to make a concept map to help them consolidate their learning.

Activity 4: Making a concept map or a mind map

  • Divide the class into smaller groups of three or four.
  • On the blackboard, write the word ‘Equations’ and put a circle around it.
  • Ask every group to think of anything that relates to equations.
  • Ask them to create a list of all such things (the more the better).
  • Ask one group to read out one of the things from their list.
  • Write it on the blackboard, connecting it to the word ‘Equations’.
  • Ask one student in each group to raise a hand if this item was on their list.
  • Ask them to delete this item from their list.
  • Ask any groups that had not written this item whether they agree that it is related to ‘Equations’.
  • Groups take it in turns to offer up other words that you add to your concept map, being careful to group words together and connect them with lines where appropriate

Case Study 4: Mrs Mehta reflects on using Activity 4

I kind of dreaded this activity because I didn’t think I fully understood how these concept maps worked, although I did look them up on the internet by searching for ‘concept maps for learning’. As all the websites proclaimed, this is a really good approach. I thought I just had to bite the bullet and have a go at this with my students.

I am sure it was far from perfect – however, it turned out that they had used this in other subjects as well (and thus knew more about it than me!). In terms of mathematics learning it did allow us to make connections, see relationships and become aware of the complexity, which is not the same as complicatedness of equations.

Pause for thought

  • When you did this activity with your class, how did it go?
  • What questions did you use to probe your students’ understanding?
  • Did you feel you had to intervene at any point?
  • What points did you feel you had to reinforce?

5 Summary

In this unit you have explored equations, looking at both their symbolic and graphical representations in order to understand the similarities and differences between different types of equations. The unit has also looked at the idea of forming an equation from a story or a picture, and forming a story from an equation. This allows students to understand that equations model the world in a way that allows solutions to be found. The use of concept maps and mind maps were also explored as tools to enable students to think around the ideas associated with equations.

Many of the ideas and techniques in this unit will work when teaching other topics. Make a note now of two topics you have to teach soon where those ideas can be used with some small adjustments.

Resources

Resource 1: NCF/NCFTE teaching requirements

The learning in this unit links to the following NCF (2005) and NCFTE (2009) teaching requirements:

  • Encourage the students capacity to construct knowledge; ensure that learning shifts away from rote methods; and to view learning as a search for meaning out of personal experiences and knowledge.
  • Organise student-centred, activity-based, participatory learning experiences.
  • See mathematics as something to talk about, to communicate through, to discuss among themselves, to work together on.
  • Help students pose and solve meaningful problems.
  • Help students use abstractions to perceive relationships, to see structures, to reason out things, to argue the truth or falsity of statements.

Resource 2: Storytelling

Stories help us make sense of our lives. Many traditional stories have been passed down from generation to generation. They were told to us when we were young and explain some of the rules and values of the society that we were born into.

Stories are a very powerful medium in the classroom: they can:

  • be entertaining, exciting and stimulating
  • take us from everyday life into fantasy worlds
  • be challenging
  • stimulate thinking about new ideas
  • help explore feelings
  • help to think through problems in a context that is detached from reality and therefore less threatening.

When you tell stories, be sure to make eye contact with students. They will enjoy it if you use different voices for different characters and vary the volume and tone of your voice by whispering or shouting at appropriate times, for example. Practise the key events of the story so that you can tell it orally, without a book, in your own words. You can bring in props such as objects or clothes to bring the story to life in the classroom. When you introduce a story, be sure to explain its purpose and alert students to what they might learn. You may need to introduce key vocabulary or alert them to the concepts that underpin the story. You may also consider bringing a traditional storyteller into school, but remember to ensure that what is to be learnt is clear to both the storyteller and the students.

Storytelling can prompt a number of student activities beyond listening. Students can be asked to note down all the colours mentioned in the story, draw pictures, recall key events, generate dialogue or change the ending. They can be divided into groups and given pictures or props to retell the story from another perspective. By analysing a story, students can be asked to identify fact from fiction, debate scientific explanations for phenomena or solve mathematical problems.

Asking the students to devise their own stories is a very powerful tool. If you give them structure, content and language to work within, the students can tell their own stories, even about quite difficult ideas in maths and science. In effect they are playing with ideas, exploring meaning and making the abstract understandable through the metaphor of their stories.

Resource 3: An example of a mind map

Figure R1.1 An example of a mind map.

Additional resources

References

Bouvier, A. (1987) ‘The right to make mistakes’, For the Learning of Mathematics, vol 7, no. 3, pp. 17–25.
Bruner, J. (1986) Actual Minds, Possible Worlds. Cambridge, MA: Harvard University Press.
Egan, K. (1986) Teaching as Story Telling: An Alternative Approach to Teaching and Curriculum in the Elementary School. Chicago, IL: University of Chicago Press.
National Council of Educational Research and Training (2005) National Curriculum Framework (NCF). New Delhi: NCERT.
National Council of Educational Research and Training (2009) National Curriculum Framework for Teacher Education (NCFTE). New Delhi: NCERT.
National Council of Educational Research and Training (2012a) Mathematics Textbook for Class IX. New Delhi: NCERT.
National Council of Educational Research and Training (2012b) Mathematics Textbook for Class X. New Delhi: NCERT.
Novak, J.D. and Gowin, D.B. (1984) Learning How to Learn. New York, NY: Cambridge University Press.
Watson, A., Jones, K. and Pratt, D. (2013) Key Ideas in Teaching Mathematics. Oxford: Oxford University Press.

Acknowledgements

Except for third party materials and otherwise stated below, this content is made available under a Creative Commons Attribution-ShareAlike licence (http://creativecommons.org/ licenses/ by-sa/ 3.0/). The material acknowledged below is Proprietary and used under licence for this project, and not subject to the Creative Commons Licence. This means that this material may only be used unadapted within the TESS-India project and not in any subsequent OER versions. This includes the use of the TESS-India, OU and UKAID logos.

Grateful acknowledgement is made to the following sources for permission to reproduce the material in this unit:

Figure 1: top left: © Rick212: http://commons.wikimedia.org/ wiki/ File:Australia_vs_India.jpg – this file is licensed under the Creative CommonsAttribution 2.0 Generic licence; top right: © Yosarian: http://commons.wikimedia.org/ wiki/ File:Indian_sweet_shop.jpg – this file is licensed under the Creative CommonsAttribution-Share Alike 3.0 Unported licence; bottom left: © Sanyambahga http://commons.wikimedia.org/ wiki/ File:Punjabi_woman_in_kitchen.jpg – this file is licensed under the Creative CommonsAttribution-Share Alike 3.0 Unported licence; bottom right: © Prattheepps http://commons.wikimedia.org/ wiki/ File:Nilgiri_ooty_railway_station_.JPG.

Every effort has been made to contact copyright owners. If any have been inadvertently overlooked the publishers will be pleased to make the necessary arrangements at the first opportunity.

Video (including video stills): thanks are extended to the teacher educators, headteachers, teachers and students across India who worked with The Open University in the productions.

 TI-AIE: Creating contexts for abstract mathematics: equations

Copyright © 200X, 200Y The Open University