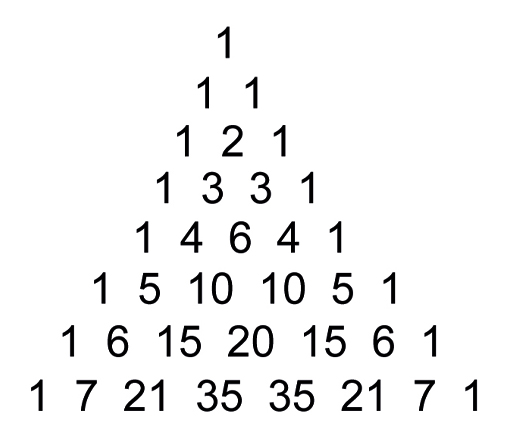
1.4 Pascal’s triangle
Remarkably, Pascal further discovered that there is a simple way of working out the division of the stake in more complex situations, e.g. if one player needs 3 points for a win and the other needs 2. The key turned out to be concealed within what today is known as ‘Pascal’s triangle’.
The triangle is constructed starting with 1, and then numbers are placed beneath it in a triangular fashion, with each number in the triangle being the sum of the two numbers immediately above it. For example: 2 is the sum of 1 + 1; 6 is the sum of 3 + 3; 15 is the sum of 10 + 5; and so it goes on indefinitely.
In the case of an interrupted game where one player needs 2 points to win and the other needs 3, you add the 2 and the 3 together to make 5. Then you look in the 5th row of the triangle, add together the first three numbers (1 + 4 + 6 = 11) and the last two (4 + 1 = 5), and divide the stake according to this proportion. So the first player will get 11/16 of the stake and the second player will get 5/16 of the stake.
In general, if player A needs m points and player B needs n points, you consult the (m + n)th row, add together the first n numbers in the row then add together the remaining m numbers in the row and divide the stake proportionally: player A gets n/(m + n) of the stake and the player B gets m/(m + n) of the stake.
Question 2
Use Pascal’s triangle to double check the solution to the game between Alex and Bill.
Answer
Alex needs 1 point; Bill needs 2 points. Look in the third row. Add the first two numbers together, 1 + 2 = 3. There is only one last number, 1. Thus Alex gets 3/4 of the stake and Bill gets 1/4 of the stake. Since the stake is £60, they get £45 and £15 respectively as before.
Pascal’s triangle was named for Blaise Pascal because of his discoveries about the triangle which he compiled together in a book, Traité du triangle arithmétique (trans. ‘Treatise on the Arithmetical Triangle’), published in 1665, three years after his death. But Pascal was not the first to study the triangle. It had been studied much earlier by mathematicians elsewhere – notably by Islamic mathematicians in the 10th century and Chinese mathematicians in the early 11th century – in connection with solving certain types of equation. In fact, Pascal’s triangle is a particularly useful mathematical tool and turns up in several areas of mathematics.
A common use of the triangle is to find what are known as the ‘combinatorial numbers’. If we have n different objects and want to choose k of them, without repetition, and the order of choice doesn’t matter, then the combinatorial number, which is written , is the number of ways in which that choice can be made. In Pascal’s triangle, using the convention that the top row of the triangle is row 0, and the first number in each row is column 0, then the number in row n and the number in column k is the number .
For example, if you’re shown 5 different plants but can choose only 3 of them, how many different combinations of plants are there for you to choose from? Or in mathematical terms, what is the value of ?
You could try writing out all the different combinations, where the plants are labelled a, b, c, d, e, then the combinations are abc, abd, abe, acd, etc., but it is much quicker to use Pascal’s triangle. You just look at the entry in the sixth row and the fourth column in the triangle (remember to start counting from zero), and you will see that the answer is 10.