1 Understanding negative numbers

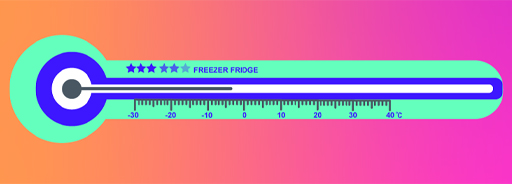
The common unit that is used to measure temperature is degrees Celsius (°C). You can check the temperature of a fridge or freezer using a special thermometer that shows the temperature between about –30 ºC and +40 ºC. Normally you don’t include the plus sign though, as it is assumed that any number without a negative sign is positive. On the thermometer in Figure 1, the marks above each of the small divisions represent one degree C. This means that the temperature recorded in Figure 1 is –4 ºC. You can see on the thermometer that the temperature increases to the right and decreases to the left.

Figure 1 A fridge thermometer

Show description|Hide description

This shows a fridge thermometer with a scale marked in one degree C intervals from –30 °C to 40 °C. The bar of mercury stops at –4 °C.

Figure 1 A fridge thermometer

You are now going to extend the work you did on number lines in Week 1 to help with understanding negative numbers.

You already know that positive whole numbers – known as natural numbers – are not sufficient to describe the world around us. Think about going to the supermarket and the various prices you see, most of these will have a decimal component showing the number of pence you have to pay. You also know that there are fraction and decimal numbers. You can show all these numbers on a number line as illustrated in Figure 2.

Figure 2 Positive numbers on a number line

Show description|Hide description

A number line from 0 to 10. One half is shown between 0 and 1, one and a half between 1 and 2.

Figure 2 Positive numbers on a number line

What happens if you extend this number line to the left? What numbers are represented in this new section? These are the negative numbers, which are all less than zero. The number line now looks like that shown in Figure 3.

Figure 3 Negative numbers on a number line

Show description|Hide description

A number line from -9 to +10. One half is shown between 0 and 1, one and a half between 1 and 2.

Figure 3 Negative numbers on a number line

Notice the order that the negative numbers are in. For example, -3 is to the left of -1. That means -3 is smaller than -1, just as in a lift, floor -3 would be lower than floor -1, and a temperature of -3 is colder than a temperature of -1.

Using the number line example gives you a useful way to start exploring these new numbers, as you’ll see in the next section.

Before you do that though, you’ll look at the convention used to show negative numbers in the rest of this course.

Throughout your work, negative numbers will be denoted by using a small subtraction sign, i.e. ‘-3’. However, when negative numbers are used in calculations they will also be enclosed in brackets, to make it clear that they are negative numbers. This is a convention that you will see in some other maths text books and material, but not all.

You may be wondering what roles negative numbers play in everyday life, and why they might be useful to get to grips with. There are a few examples to look at in the next section.