3.3 Areas of circles

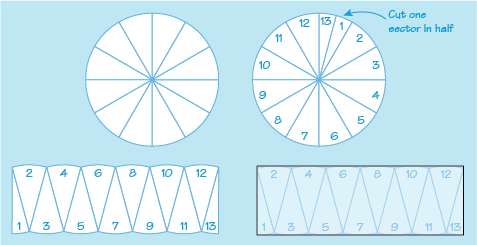
You can show that the area of a circle is calculated by multiplying the square of the radius by , by splitting the circle into equal sized segments and then arranging these to form a rectangle as shown in Figure 25:

Figure 25 Circle area by sectors

Show description|Hide description

This shows two circles, which are the same size. The 1^st is divided into 12 equally sized segments. The 2^nd is divided into 12 equally divided segments as well but the 1^st segment has been divided into two, to create two half segments. The segments are numbered from 1 to 13.

Under the circles the segments are shown fitted together to form a rectangular like shape. The two half segments form the ends of the rectangle and circular edges of the segments the top and bottom of the rectangle. Next to this a rectangle has been drawn around this same shape formed by the segments. Each segment again is numbered from 1 to 13.

Figure 25 Circle area by sectors

If each segment is made gradually smaller, any ‘bumps’ along the top and bottom edges will be smoothed out to form a line that is closer and closer to being straight. The height of the rectangle will then be the same as the radius of the circle and the length half of the circumference. The area of this rectangle will therefore be equivalent to that of the circle.

The

The circumference and radius are related by the following formula:

So

So putting this together gives:

The formula for the area of a circle is therefore:

If you are given the diameter of a circle instead of the radius, the first step to take when working out the area would be to halve the diameter.

See how you get on with applying this new formula in the next activity.

The last few sections have included a number of different formulas, so you might appreciate a quick summary to bring them altogether. You might also like to take a note of them alongside any new vocabulary you’ve come across

From your study of measurement in Week’s 1 and 2 of the course you will know that there is one final property of shapes that hasn’t been covered, that is capacity or volume. So, you’ll move onto that now in the final part of this week’s study.