Week 2 Identifying tricky topics
Introduction
As you saw in Week 1, tricky topics are the practical application of threshold concepts and their theoretical underpinning. Your understanding of tricky topics will be developed over weeks 2 and 3 by identifying and breaking down tricky topics in practice and the tricky topic process. You will learn the many ways in which you can identify tricky topics and the problems that students have in understanding them. You will know many ways of finding out why students have problems with them. You will then be in a position to begin to think about how to design learning to specifically overcome them (in Week 4).
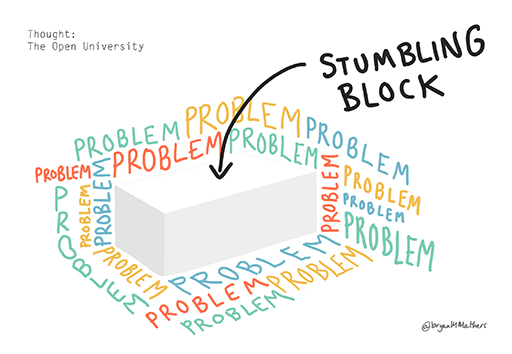
In this week, you will consider what makes tricky topics tricky. Why are these topics so difficult to learn and teach? This week you will see how tricky topics are made up of several assessable parts (which are referred to as stumbling blocks) and how each of the stumbling blocks can be identified and constructed from students’ specific problems in the topic. The students’ problems (which are referred to as problem examples) highlight their misunderstanding of the tricky topic in general and may identify one or two of the stumbling blocks in particular. You will learn how the students’ problem examples can be grouped together to form stumbling blocks or visa-versa, and how stumbling blocks can be defined by many problem examples.
By the end of this week you should be able to:
- identify tricky topics and their key components
- conduct a mini ‘needs analysis’ to identify students’ problems in tricky topics
- use the ‘problem distiller’ to examine why students have these problems
- begin to appreciate the relationship between stumbling blocks and students’ problem examples.