3 Negative numbers
Negative numbers come into play in two main areas of life: money and temperature. Watch the animations below for some examples.
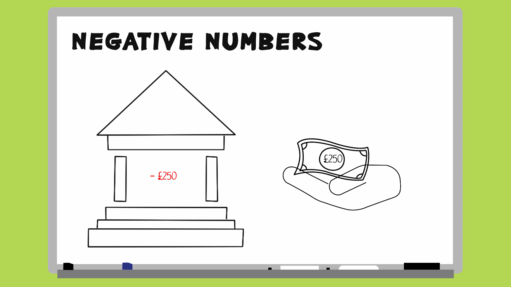
Transcript
Negative numbers come into play in two main areas of life: money and temperature. If your bank balance is showing as -£250, this means that your balance is £250 below zero. In other words, you owe the bank £250.
Similarly, you'll be used to seeing winter weather forecasts predicting temperatures of -10 degrees Celsius. This means the temperature will be 10 degrees below zero degrees Celsius.
It can be useful to think of a thermometer if you're trying to find the difference between two numbers, where one or both of the numbers are negative. If the temperature is increasing, you go up the thermometer. If the temperature is decreasing, you travel down the thermometer.
For example, you'd like to know the answer to the sum [6 - 10]. Starting at number 6 on a thermometer and travelling down, it's easy to see that subtracting 10 will result in a number less than zero.
What is the answer to the sum [-5 + 12]? This time, we're starting below zero and travelling up the thermometer by 12 places, giving an answer of 7.
Can you work out the answer to the sum [-1 - 8]? In this example, we start below zero and the answer is also below zero: -9. Now try the next activity for more practise with negative numbers.
Activity 6: Negative and positive temperature
- The table below shows the temperatures of cities around the world on a given day.
| London | Oslo | New York | Kraków | Delhi |
|---|---|---|---|---|
| 4˚C | −12C | 7˚C | −3˚C | 19˚C |
- a.Which city was the warmest?
- b.Which city was the coldest?
- c.What is the difference in temperature between the warmest and coldest cities?
Answer
a.Delhi was the warmest city as it has the highest positive temperature.
b.Oslo was the coldest city as it has the largest negative temperature.
c.The difference between the temperatures in these cities is 31˚C.
From 19˚C down to 0˚C is 19˚C and then you need to go down a further 12˚C to get to −12˚C.
- Look at this bank statement.
- a.On which days was Sonia Cedar overdrawn, and by how much?
- b.How much money was withdrawn on 11 October?
- c.How much was added to the account on 15 October?
Answer
- a.The minus sign (−) indicates that the customer is overdrawn, i.e. owes money to the bank.
The amount shows how much they owe. So Sonia Cedar was overdrawn on 11 October by £20 and by £50 on 21 October.
- b.£120 was withdrawn on 11 October.
The customer had £100 in the account and must have withdrawn another £20 (i.e. £100 + £20 = £120 in total) in order to have a £20 overdraft.
- c.The customer owed £20 and is now £70 in credit, so £90 must have been added to the account.
- a.The minus sign (−) indicates that the customer is overdrawn, i.e. owes money to the bank.
You have now learned how to use all four operations and how to work with negative numbers. Every other mathematical concept hinges around what you have learnt so far; so once you are confident with these, you can do anything! Fractions, for example, are linked very closely to division and multiplication. Let’s put your newly found skills to good use in the next section, which deals with fractions.
Summary
In this section you have:
- learned the two main contexts in which negative numbers arise in everyday life – money (or debt!) and temperature
- practised working with negative numbers in these contexts.

