1.1 Additive versus multiplicative thinking
For many young learners, comparisons are described in additive terms and groups are compared used subtractive language.
This form of reasoning is based on additive thinking.
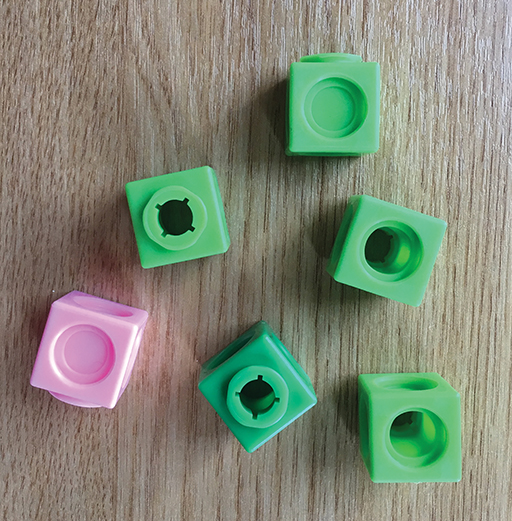
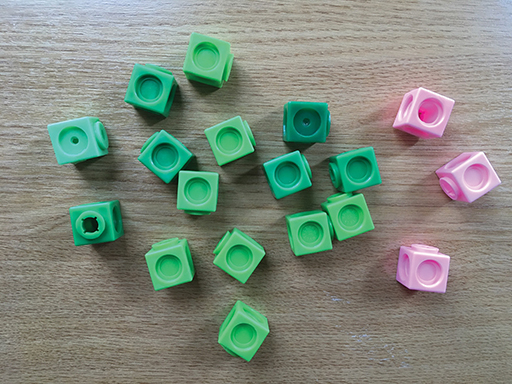
For example, in both images in Figures 2 and 3 there are some green and pink cubes. The ratio of green cubes to pink cubes, expressed as G : R, remains the same in each picture.
If a learner is using additive thinking, they will describe the change from 5 to 15 green cubes as an addition of 10, and the change from 1 to 3 pink cubes as an addition of 2.
When a learner uses multiplicative thinking, they will describe the same changes as multiplying by 3.
Being able to describe proportional situations using multiplicative language is an indicator of proportional reasoning.
Activity 1 Growing dogs
Activity 2 Reflecting
Consider the problem above. How do you think learners may respond to this question?
Discussion
If learners think in absolute terms, or using additive thinking, they may suggest that Ralph has grown more because his weight has increased by 4 kg whereas Ronnie’s weight has increased by only 3 kg.
If learners think in relative terms, or using multiplicative thinking, they may suggest that Ronnie has grown more because his weight has more than doubled and Ralph’s weight has less than doubled.
Activity 3 Speed on a cycling track
Activity 4 Reflecting
How did you approach the task in Activity 3?
Discussion
It is easy to fall into the trap here of taking a proportional approach, resulting in Isaac completing 5 laps, when actually this task requires additive reasoning.
The key words here are ‘cycling equally fast’. Ava and Isaac are travelling at the same speed, so it is not a proportional relationship. Ava had a head start, so completed an extra 6 laps, meaning that when Ava has completed 15 laps, Isaac will have completed 9 laps.