3.1 Doing some doing and undoing
Now you’ve had a look at an example, see how you get on with the next activity.
Activity 7 Working out the number
Try to work out what number was thought of in the following problems. You may find it helpful to use some doing-undoing diagrams to visualise, and keep track of these.
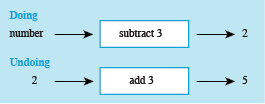
- a.Think of a number, subtract 3, and the answer is 2.
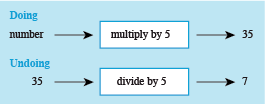
- b.Think of a number, multiply by 5, and the answer is 35.
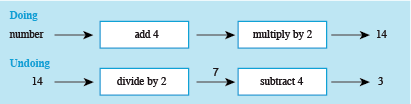
c.Think of a number, add 4, double it, and the answer is 14.
Click on ‘reveal comment’ if you would like a hint
Comment
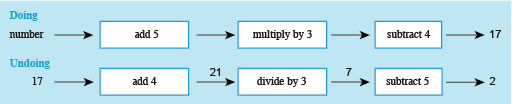
When you have to deal with more than one operation, just take each step in turn. Write down the doing diagram and draw the undoing one underneath, working backwards to undo each operation.
- d.Think of a number, add 5, multiply by 3 and subtract 4. The answer is 17.
You may be wondering what is the point of all this doing/undoing business! Sometimes there is a need to change a formula so that you can work out something different from it. This is called rearranging the formula and does occur quite frequently in any subject area that uses formulas. The same technique that you have been looking at in this section on ‘undoing’ instructions can be used when rearranging formulas, as you’ll see in the next section.