2.2 Generalising with sequences

Sequences also give learners a context in which to generalise, particularly when they are defined using diagrams or shapes. If you look at sequence resources you will find two main approaches to generalising activities. We can describe these as looking for changes versus looking for structure. Many textbooks written in the 2000s focus on the first approach, while more recent research (Kieran et al., 2016) suggests that learners also need to look for structure. The next two activities in this section exemplify each approach.

In the first approach, the intention is for learners to observe a pattern of change across a sequence of examples by asking ‘What is the same and what is different?’

Activity 5 Looking for changes

Timing: Allow 10 minutes

Each term of the sequence in Figure 7 is a house. Draw the next two terms.

What is the same and what is different about the houses?

What would the 26th house look like?

Figure 7 A sequence of houses

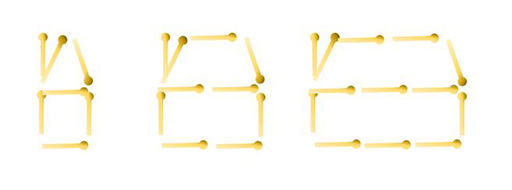
How many matches are needed for each of the houses? Complete the table below to make a number sequence.

Then describe a method for finding how many matches are needed for house n.

Position	1	2	3	4	10	26
Number of matches

Discussion

Did you continue the pattern by looking at the changing shapes?

Or by thinking about numbers – how many matches you would need?

You will explore each of these ways of finding the n^th term. (This is the position-to-term rule.)