3 Using number lines to develop understanding of positive and negative numbers
A number line such as Figure 1 is a geometrical idea that can be imagined as a set of points arranged in particular order on a straight line. A mathematical line has infinite length and is infinite in mutually opposite directions, but it is always centred at the origin, or zero. A number line can help students make sense of negative numbers and begin to understand adding and subtracting them.
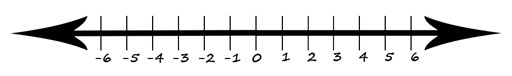
A number line can be so useful that it is a good idea to construct and display a large line divided into equally spaced intervals in any classroom where mathematics is learned, as in Figure 2.
Making the line in such a way that the numbers it represents can be written or attached separately will mean that it can be used for thinking about any part of the number system. Each division can then represent:
- units, tens or hundreds, etc.
- fractions or decimals, including very small decimals
- standard form
– as well as many other mathematical ideas.
Once students are used to seeing a number line on a wall or on their desks, they will be able to imagine the line in order to check their reasoning.
The idea of a negative number only has existence in relation to positive numbers using zero as the origin. That is, a point is selected on a number line and zero is assigned to it so that one side of zero is positive and the other negative. In order to help students think in terms of opposites, it is conventional to use the right-hand side of a horizontal line to represent positive numbers and the left-hand side to represent negative numbers. However, it is also a good idea to also use a vertical line, where the numbers above zero are represented by positive numbers and the negative numbers are below zero.
Whether using a horizontal or a vertical line, moving the point that is assigned to zero can help students understand that this is just a part of an infinite line, and that this part is being considered in lessons on negative numbers because zero is where things change.
The following activity uses a number line drawn on a blackboard in a way that will help the students think about how to use negative numbers and how to add and subtract those numbers. The activity also uses the expression ‘Imagine if …’. This expression can help the students to use their imagination and not be limited by their belief that mathematics can only be ‘right’ or ‘wrong’. Knowing this is especially important in mathematical modelling (such as in word problems) where a model represents a perceived situation, which is not necessarily valid in all cases or may not even reflect a true real-life situation (Bruner, 1986).
Activity 2: Learning from misconceptions and mistakes
Part 1: How positive was that?
Draw a number line on the blackboard going from –10 to 10. Ask the students to imagine positive things that can happen and ask them to imagine where they would put that on the number line. For example, ‘Someone gives me Rs. 10’ is a little positive; ‘Someone gives me Rs. 100’ is more positive.
Then ask them to suggest negative things, for example, ‘My new outfit was splashed with mud when a rickshaw went past and I didn’t notice’, or ‘My cricket team lost a match’. Each time ask them to imagine where to put the idea on the number line, asking them to imagine ‘How positive do you feel?’ or ‘How negative was that?’
Part 2: The ‘happiness’model
The ideas in Part 1 can then be extended to adding and subtracting negative numbers.
Say to the class:
I feel OK today; imagine I score 2 (pointing to the number line) on this happiness scale.
Imagine if someone gave me nine sweets (a positive!), how would I feel then? Yes, I could move up 4 to 6.
Now imagine if someone told me I had to stay after school (negative) how would I feel then? Yes, down 1, to 5.
Imagine if you took away seven of my chocolates? How would I feel? Sadder? Yes, I need to go down 7, to –2.
What if you told me I could go home early?
…
Adding something positive or taking away something negative improves the situation (go up the number line).
Adding something negative or taking away something positive makes the situation worse (go down the number line).
(Source: Part 2 was adapted from NRICH, undated.)
Video: Talk for learning |
Case Study 2: Mrs Agarwal reflects on using Activity 2
I used the ideas in Activity 2 to explain positive and negative numbers to my class. Before I started I said, ‘I believe that adding and subtracting with negative numbers makes sense’.
I wrote a big number line along the top of my blackboard. With the students, I brainstormed on ‘Things that are POSITIVE’ and ‘Things that are NEGATIVE’. We talked for quite a while about how you feel if someone gives you a positive thing, or if someone takes one away. We also talked about how you feel if someone gives you a negative thing, or if someone takes one away.
Then we went on to use the happiness model. I went through getting sweets and losing my sweets, pointing to where I was on the happiness scale and then writing down the mathematical expression of what I was saying. I asked several of the students to tell their own story using the scale and to start with I wrote the sums as they were telling the story.
I then asked the students to work in groups of three or four. They drew a number line in chalk on their desks then one told a story while another pointed to where they were on the number line and another wrote the addition and subtractions that they were doing. I have never seen so many smiles!
Pause for thought
|
2 The need for negative numbers
