2 The role of choice
Playfulness is considered important to support creativity because in play you explore many possible solutions in a spontaneous way. This is known as divergent thinking. The word ‘playfulness’ is often associated with young children, but should not be restricted to them. Play is about exploring and experimenting, which anyone of any age can do. Simply watching children play can be a good reminder of their creativity.
When they are exploring and experimenting, it is important that students have choices: the choice to approach a problem in different ways, the option to make mistakes or the choice to come up with their own conjectures and test whether they are valid or not. In Activity 1 you give students that choice by simply asking the question, ‘In how many ways can you …?’
The activity aims for students to become knowledgeable and confident that closed polygons can be divided into right-angled triangles. This will enable them to ‘just do it’ when they have to find right-angled triangles to use in trigonometry problems later, such as when proving the cosine rule. In that way, being able to play with right-angled triangles in a polygon can become a tool to ‘getting unstuck’ later.
This task works well for students first exploring possibilities on their own, and then discussing these with classmates or in groups to get more ideas and refine their thinking.
Before attempting to use the activities in this unit with your students, it would be a good idea to complete all (or at least part) of the activities yourself. It would be even better if you could try them out with a colleague, as that will help you when you reflect on the experience. Trying the activities yourself will mean that you get insights into learners’ experiences that can in turn influence your teaching and your experiences as a teacher. When you are ready, use the activities with your students. After the lesson, think about the way that the activity went and the learning that happened. This will help you to develop a more learner-focused teaching environment.
Activity 1: Students investigate triangles in polygons
Ask your students the following:
- In how many ways can you divide each of the shapes in Figure 1 into right-angled triangles?
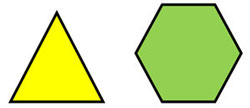
- Draw any right-angled triangle (Figure 2). What kind of closed polygons can you construct by using this triangle as a building block?

Figure 3 shows an example.

- Do you think that all closed polygons can be formed by right angled triangles? Give reasons to justify your answer.
- Why do you think this activity asks you to investigate whether any closed polygons can be made up of right-angled triangles?
You may also want to have a look at the key resource ‘Involving all [Tip: hold Ctrl and click a link to open it in a new tab. (Hide tip)] ’.
Case Study 1: Mrs Nagaraju reflects on using Activity 1
This is the account of a teacher who tried Activity 1 with her secondary students.
What really struck me was the enthusiasm of the students when doing this activity. I had not expected that. We read the first question of the task together and I then asked the students to work on their own for a while, because I wanted them to have the opportunity to think on their own first. They could discuss their ideas with their classmates when they felt ready.
Everyone got really busy trying to divide the given figure into right-angled triangles. Some started by dividing the figure into triangles that were not right-angled triangles; I decided not to interfere immediately, as I would normally do, but allow the students to make their own mistakes! I noticed that most of these students self-corrected: they glanced at other students’ work, read the question again and changed what they were doing. They did not lose their enthusiasm and continued with what seemed like new energy.
When some students had started discussing their ideas, I stopped the class and asked, ‘Why do you think it is important to work with right-angled triangles?’ I asked several of the students who had done it ‘wrong’ to talk about what they had experienced and what they thought. In this way, everyone in the class learned that making mistakes actually can offer very good learning opportunities.
They found making the closed polygons with right-angled triangles even more exciting, because they had the freedom to form figures of their own. Again, some students ended up making non-closed polygons but self-corrected. Gaurav made cut-outs of right-angled triangles and used these to compile different figures. Some were known geometric figures such as hexagons; others were more random formations and some were even figurines existing of several closed polygons. Students were interested in each other’s work and were inspired by what they saw their peers doing, and used similar approaches. Others used straight edges and a pencil to draw.
Look at Resource 2, ‘Monitoring and giving feedback’, for further information.
Reflecting on your teaching practice
When you do such an activity with your class, reflect afterwards on what went well and what went less well. Consider the questions that led to the students being interested and being able to get on and those where you needed to clarify. Such reflection always helps with finding a ‘script’ that helps you engage the students to find mathematics interesting and enjoyable. If they do not understand and cannot do something, they are less likely to become involved. Use this reflective exercise every time you undertake the activities, noting, as Mrs Nagaraju did, some quite small things that made a difference.
Pause for thought Good questions to trigger such reflection are:
|
1 Creativity in learning mathematics
